
Find the coordinates of point A, where AB is the diameter of a circle where the center is (2, -3) and B is the point (1, 4).
Answer
511.5k+ views
4 likes
Hint: This is a simple question based on the mid-point theorem. In any straight line AB, whose coordinates are
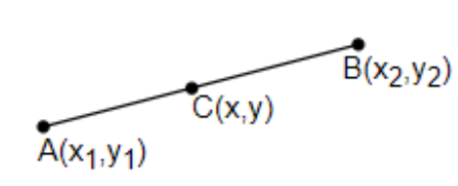
We will use this concept and find the coordinates of point A with the other values given to us.
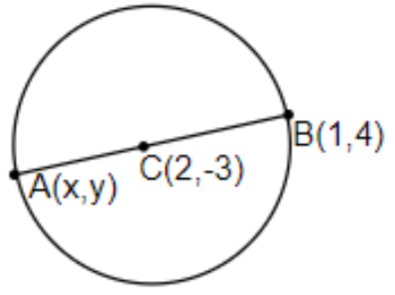
In this question, AB is the diameter of a circle and the center is the mid-point of any diameter, which is given as (2, -3), and the coordinates of B are given as (1, 4). It can be represented as in the figure below.
Let us assume the coordinate of A as
Similarly, we will find the value of y also,
Therefore, we get the coordinates of A as (3, -10).
Note: This question is easy to solve but it also has places for silly mistakes, like we have to remember that we have to add the x-coordinates of the endpoints and divide by 2 to get the coordinates of the mid-point. We have to do similarly with the y-coordinates too. The formula for x-coordinate of mid-point is

We will use this concept and find the coordinates of point A with the other values given to us.

In this question, AB is the diameter of a circle and the center is the mid-point of any diameter, which is given as (2, -3), and the coordinates of B are given as (1, 4). It can be represented as in the figure below.
Let us assume the coordinate of A as
Similarly, we will find the value of y also,
Therefore, we get the coordinates of A as (3, -10).
Note: This question is easy to solve but it also has places for silly mistakes, like we have to remember that we have to add the x-coordinates of the endpoints and divide by 2 to get the coordinates of the mid-point. We have to do similarly with the y-coordinates too. The formula for x-coordinate of mid-point is
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




