
Find the center of mass of a uniform semi-circular ring of radius R.
Answer
446.2k+ views
3 likes
Hint: The center of mass of a regular uniform object can be found by finding the center of mass of a point infinitesimal mass and integrating this for the whole object. This can be done by finding out a relation between the mass and another variable in the body with respect to which the relation can be integrated for the whole body to get the center of mass of the whole body.
Formula used:
The center of mass
where
Complete step-by-step answer:
Let us first draw a diagram for the ring along with proper coordinates.
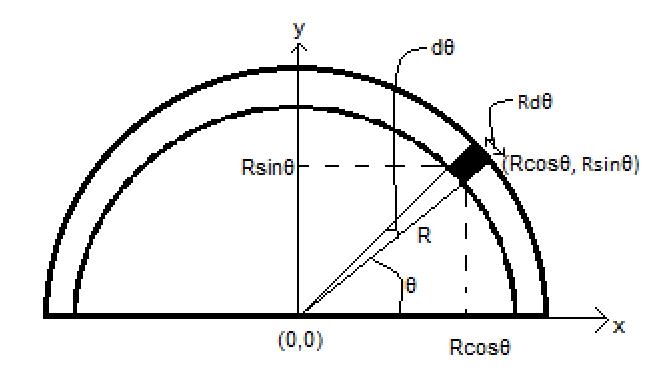
We will take the origin and the axes as shown in the figure.
Since the ring is uniform, it has a constant mass per unit length
Let the total mass of the Ring be
We will take an infinitesimal strip of mass
Therefore, using the definition of
Now, the center of mass
We will take the reference point as the origin
Hence, we will find the center of mass by using (3) and integrating the small strip to cover the whole length of the ring. The mass of the strip is
Therefore, using (3)
Similarly,
Hence,
Hence, the center of mass of a semi circular ring lies on the vertical passing through its center of curvature at a distance of
Note: It can also be noted that since the semi circular ring is symmetric about the vertical y-axis, the abscissa of the center of mass lies on the vertical axis itself. Thus, there was no need to find
Formula used:
The center of mass
where
Complete step-by-step answer:
Let us first draw a diagram for the ring along with proper coordinates.

We will take the origin and the axes as shown in the figure.
Since the ring is uniform, it has a constant mass per unit length
Let the total mass of the Ring be
We will take an infinitesimal strip of mass
Therefore, using the definition of
Now, the center of mass
We will take the reference point as the origin
Hence, we will find the center of mass by using (3) and integrating the small strip to cover the whole length of the ring. The mass of the strip is
Therefore, using (3)
Similarly,
Hence,
Hence, the center of mass of a semi circular ring lies on the vertical passing through its center of curvature at a distance of
Note: It can also be noted that since the semi circular ring is symmetric about the vertical y-axis, the abscissa of the center of mass lies on the vertical axis itself. Thus, there was no need to find
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

What organs are located on the left side of your body class 11 biology CBSE

A car travels 100 km at a speed of 60 kmh and returns class 11 physics CBSE

Name the Largest and the Smallest Cell in the Human Body ?

Explain zero factorial class 11 maths CBSE

Where can free central placentation be seen class 11 biology CBSE




