
How do you find the base of a right triangle when given the hypotenuse is 14 ft. The angle formed between the hypotenuse and base is 41 degrees?
Answer
470.4k+ views
1 likes
Hint: First assume the angle formed between the hypotenuse and base as ‘
Complete step by step solution:
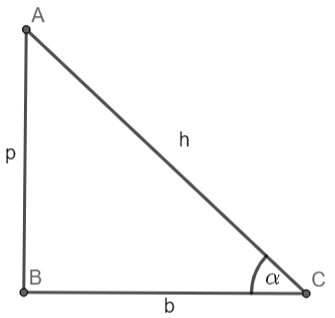
ABC is a right angle triangle with base ‘b’, perpendicular ‘p’ and hypotenuse ‘h’.
Given, the hypotenuse is 14 ft. and the angle formed between the hypotenuse and base is 41 degrees.
Let the angle formed between the hypotenuse and base is ‘
So, h=14 ft. and
We know, in right angle triangle ABC
Putting the values of angle ‘
Hence the base is 10.566 ft.
This is the required solution of the given question.
Note: Since now we have both the values of hypotenuse and base, we can also find the perpendicular using Pythagoras' theorem. For the above triangle Pythagoras' theorem can be applied as
Putting the values of ‘h’ and ‘b’ in
Complete step by step solution:

ABC is a right angle triangle with base ‘b’, perpendicular ‘p’ and hypotenuse ‘h’.
Given, the hypotenuse is 14 ft. and the angle formed between the hypotenuse and base is 41 degrees.
Let the angle formed between the hypotenuse and base is ‘
So, h=14 ft. and
We know, in right angle triangle ABC
Putting the values of angle ‘
Hence the base is 10.566 ft.
This is the required solution of the given question.
Note: Since now we have both the values of hypotenuse and base, we can also find the perpendicular using Pythagoras' theorem. For the above triangle Pythagoras' theorem can be applied as
Putting the values of ‘h’ and ‘b’ in
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
Explain why it is said like that Mock drill is use class 11 social science CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE




