
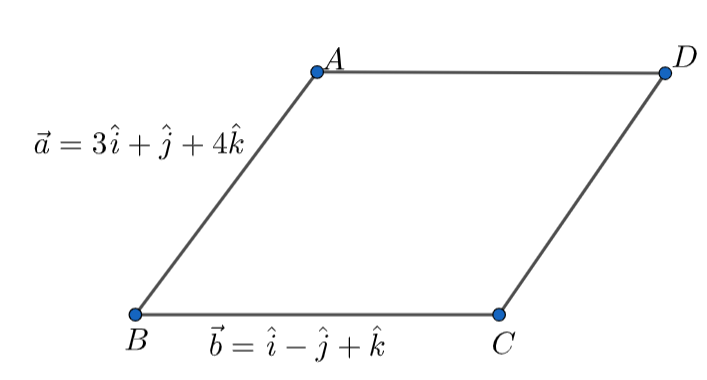
Find the area of a parallelogram whose adjacent sides are given by the vectors
Answer
489k+ views
Hint: The adjacent side vectors of the parallelogram ABCD are
Complete step by step answer:
According to the question, we are given the adjacent sides of a parallelogram in vector form and we are asked to find the area of the parallelogram.
The vector form of side AB of the parallelogram is
The vector form of side BC of the parallelogram is
Here, we have to find the area of the parallelogram.
We know the formula that if the vectors
Now, from equation (1), equation (2), and equation (3), we get
The area of the parallelogram =
We know the property that
Now, from equation (4) and equation (5), we get
The area of the parallelogram ABCD =
We know the formula for the magnitude of a vector
Now, from equation (6) and equation (7), we get
The area of the parallelogram =
Therefore, the area of the parallelogram is
Note:
We can also solve this question using the matrix method formula that is, the area of the parallelogram whose adjacent side vectors are
Complete step by step answer:
According to the question, we are given the adjacent sides of a parallelogram in vector form and we are asked to find the area of the parallelogram.
The vector form of side AB of the parallelogram is
The vector form of side BC of the parallelogram is
Here, we have to find the area of the parallelogram.
We know the formula that if the vectors

Now, from equation (1), equation (2), and equation (3), we get
The area of the parallelogram =
We know the property that
Now, from equation (4) and equation (5), we get
The area of the parallelogram ABCD =
We know the formula for the magnitude of a vector
Now, from equation (6) and equation (7), we get
The area of the parallelogram =
Therefore, the area of the parallelogram is
Note:
We can also solve this question using the matrix method formula that is, the area of the parallelogram whose adjacent side vectors are
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




