
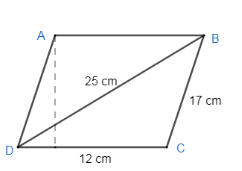
Find the area of a parallelogram given in Figure. Also find the length of the altitude from vertex A on the side DC. (in

Answer
525k+ views
Hint: To find the area of a parallelogram
Complete step-by-step answer:
We know that the diagonal of a parallelogram divides it into two congruent triangles.
So, area of a parallelogram
Here, we will find the area of
According to Heron’s formula the area of a triangle with sides a, b, & c is given as –
Where,
Here, we have from the figure –
So, we will get the value of s as –
Now, we will put the value of a, b, c and s in the formula
Since ABCD is a parallelogram,
Now, we will find the area of parallelogram.
Area of a parallelogram
Next we will find the altitude of a parallelogram be h.
Let us consider the altitude of a parallelogram to be ‘h’.
Also, area of a parallelogram
We know that the altitude of a parallelogram is also known as Height of a parallelogram.
Therefore,
Height of altitude from vertex A on side CD of the parallelogram
Hence, the area of a parallelogram for the given figure is
Note: We have taken the area of both triangles as the same here, but if a student doesn't know that, they can find it separately and then add them together. The mistake of finding an area of parallelogram as
Complete step-by-step answer:
We know that the diagonal of a parallelogram divides it into two congruent triangles.
So, area of a parallelogram
Here, we will find the area of
According to Heron’s formula the area of a triangle with sides a, b, & c is given as –
Where,
Here, we have from the figure –

So, we will get the value of s as –
Now, we will put the value of a, b, c and s in the formula
Since ABCD is a parallelogram,
Now, we will find the area of parallelogram.
Area of a parallelogram
Next we will find the altitude of a parallelogram be h.
Let us consider the altitude of a parallelogram to be ‘h’.
Also, area of a parallelogram
We know that the altitude of a parallelogram is also known as Height of a parallelogram.
Therefore,
Height of altitude from vertex A on side CD of the parallelogram
Hence, the area of a parallelogram for the given figure is
Note: We have taken the area of both triangles as the same here, but if a student doesn't know that, they can find it separately and then add them together. The mistake of finding an area of parallelogram as
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which of the following districts of Rajasthan borders class 9 social science CBSE

What is 85 of 500 class 9 maths CBSE

What is the full form of pH?

Define human made resources

India has states and Union Territories A 29 7 B 28 class 9 social science CBSE

What is the importance of natural resources? Why is it necessary to conserve them?




