
Explain the geometrical meaning of angular momentum.
Answer
443.5k+ views
Hint: The angular momentum is considered to be the rotational analogue of the linear momentum. The angular momentum of a body is defined as the cross product of the position of the body relative to the origin and the linear momentum of the body. To obtain the geometrical interpretation of the angular momentum we can represent the position vector of the body graphically as it rotates about a given axis.
Formulae used:
The angular momentum of a body is given by,
The linear momentum of a body is given by,
Complete step by step solution:
Step 1: Sketch a graph depicting the linear momentum vector and the position vector of a particle rotating in an anticlockwise direction.
We consider a particle that rotates in the XY plane about the z-axis as shown in the figure sketched below.
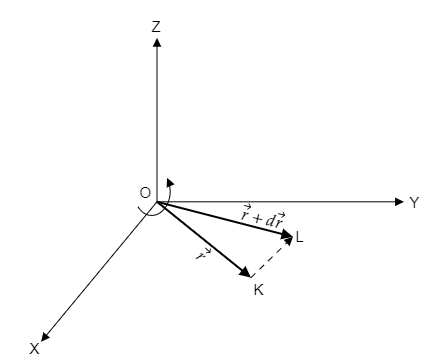
In the above figure, at any instant of time
As the particle rotates, the position vector of the particle will sweep an area
Step 2: Express the area swept by the position vector in the small interval of time.
The displacement of the particle in the small interval of time
From the above figure, the area swept by the position vector in the time
Dividing equation (1) by
Now we know that the first derivative of the position vector with respect to time corresponds to the velocity vector of the particle i.e.,
So the area swept by the position vector per unit time becomes,
The angular momentum of the particle is expressed as
Substituting equation (3) in (2) we get,
In equation (4), the term
So from equation (4), we can conclude that the angular momentum of a particle about a given axis will be twice the product of the mass of the particle and the areal velocity of the position vector of the particle. This is considered to be the geometrical interpretation of the angular momentum.
Note: Here the area swept by the position vector is given by the area of the triangle OKL whose base is the displacement vector
Formulae used:
The angular momentum of a body is given by,
The linear momentum of a body is given by,
Complete step by step solution:
Step 1: Sketch a graph depicting the linear momentum vector and the position vector of a particle rotating in an anticlockwise direction.
We consider a particle that rotates in the XY plane about the z-axis as shown in the figure sketched below.

In the above figure, at any instant of time
As the particle rotates, the position vector of the particle will sweep an area
Step 2: Express the area swept by the position vector in the small interval of time.
The displacement of the particle in the small interval of time
From the above figure, the area swept by the position vector in the time
Dividing equation (1) by
Now we know that the first derivative of the position vector with respect to time corresponds to the velocity vector of the particle i.e.,
So the area swept by the position vector per unit time becomes,
The angular momentum of the particle is expressed as
Substituting equation (3) in (2) we get,
In equation (4), the term
So from equation (4), we can conclude that the angular momentum of a particle about a given axis will be twice the product of the mass of the particle and the areal velocity of the position vector of the particle. This is considered to be the geometrical interpretation of the angular momentum.
Note: Here the area swept by the position vector is given by the area of the triangle OKL whose base is the displacement vector
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
Explain why it is said like that Mock drill is use class 11 social science CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE




