Question

Why do the electrostatic field lines not form a closed loop?

Answer 1

Hint: to give the answer of this question, we use the basic theory related to the conservative field. As we know, an electric field line shows the direction of the force on a small positive test charge. The test charge does not tend to return to its starting point so the field line doesn’t form a closed loop.

Complete Step-by-Step solution:
A time varying magnetic field can produce an electric field with a closed loop which in turn can produce a magnetic field and the process goes on resulting in propagation of electromagnetic waves. But, electric field lines due to static charges, by definition, start from positive charge and end at negative charge or end at infinity where negative charge is supposed to be existing
                                                                 

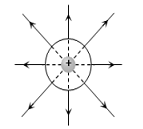
For example, if we have positive charge, then its electric field lines are radially outward direction as shown in the above figure.
In Maxwell’s equations we have Div. of B is zero as there is no magnetic monopole. But, Div. of E is not equal to zero as there we have electric monopoles. Closed field lines lead to Div. of the field=0.
Electric field lines start (or diverge) from the +ve (positive) charge and terminate (or converge) on negative charge. If the electric field lines form a closed loop, these lines must originate and terminate on the same charge which is not possible because electric field lines always move from positive to negative.
The main reason behind the electrostatic field lines not forming a closed loop is that the no electric field line exists inside the charged body.
Therefore, we say electrostatic field lines never form closed loops.

Note- Electric fields are said to be conservative vector fields, meaning you can derive an expression for the field by taking the gradient of a potential function. Gradient is a differential operator that just tells you the direction of greatest increase of a function. If an electric field has closed loops, that means the function continually increases around that loop, which doesn't make sense. A vector field with closed loops is a characteristic of magnetic fields. Those are nonconservative vector fields.