
Draw the graph of
Answer
480k+ views
1 likes
Hint: Here, we will first find the points of the graph by substituting some value of
Complete step-by-step answer:
The given equation is
We observe from this equation that the powers of
The graph of a linear equation is always a straight line.
We will first find two points lying on the graph of the given linear equation. These two points should satisfy the given linear equation.
Let us rewrite the given equation as
Now we will substitute
We see that when
To find another point, we will put
Adding the terms, we get
In this case, we get
So, the second point is
Using these points, we will draw the graph of
The point
Therefore, we get the graph as follows:
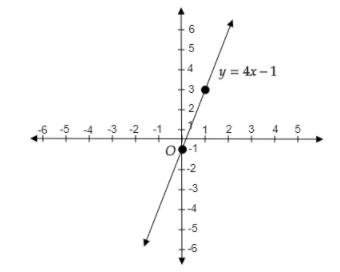
Note: Another method to draw the graph is by slope-intercept form.
We shall compare the given linear equation to the slope-intercept form of a linear equation. The slope-intercept form
Comparing the equation
Here the slope is 4 and the
First, we have to mark the
Since, the
Now, the slope is 4, which can be written as
Here the numerator 4 means we have to go 4 units up the point
So, the point we reach is
Complete step-by-step answer:
The given equation is
We observe from this equation that the powers of
The graph of a linear equation is always a straight line.
We will first find two points lying on the graph of the given linear equation. These two points should satisfy the given linear equation.
Let us rewrite the given equation as
Now we will substitute
We see that when
To find another point, we will put
Adding the terms, we get
In this case, we get
So, the second point is
Using these points, we will draw the graph of
The point
Therefore, we get the graph as follows:

Note: Another method to draw the graph is by slope-intercept form.
We shall compare the given linear equation to the slope-intercept form of a linear equation. The slope-intercept form
Comparing the equation
Here the slope is 4 and the
First, we have to mark the
Since, the
Now, the slope is 4, which can be written as
Here the numerator 4 means we have to go 4 units up the point
So, the point we reach is
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
Explain why it is said like that Mock drill is use class 11 social science CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE




