
How do you draw the graph of
Answer
475.5k+ views
Hint:We are required to draw the graph of the function given to us,
Complete step by step solution:
In this question, we are required to draw the graph of the function
So,
Rearranging the terms and grouping the terms with x and y, we get,
Adding and subtracting same terms from the expression, we get,
Now, forming whole square terms and simplifying calculations, we get,
Now, we can conclude that the given equation is of a circle as it resembles the standard equation of a circle.
Now comparing with standard form of equation of circle
So, in the given equation, we get the coordinates of center
Plotting the graph of the equation, we get,
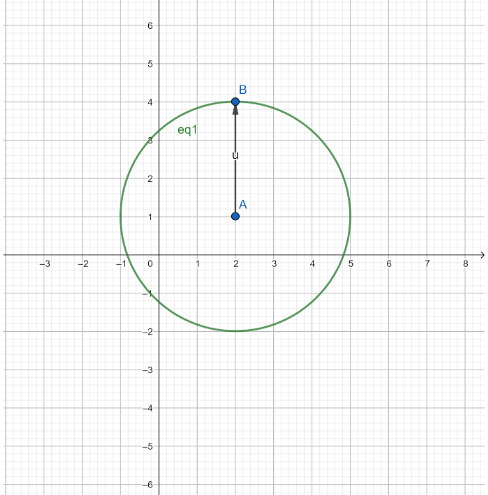
Note: To draw the graph of a function, we either need the points that lie on the graph of the function when plotted on graph paper or the equation of the function. In the given question, we are given an equation of circle to plot on the graph that can be plotted easily once we get to know the center of the circle and the radius.
Complete step by step solution:
In this question, we are required to draw the graph of the function
So,
Rearranging the terms and grouping the terms with x and y, we get,
Adding and subtracting same terms from the expression, we get,
Now, forming whole square terms and simplifying calculations, we get,
Now, we can conclude that the given equation is of a circle as it resembles the standard equation of a circle.
Now comparing with standard form of equation of circle
So, in the given equation, we get the coordinates of center
Plotting the graph of the equation, we get,

Note: To draw the graph of a function, we either need the points that lie on the graph of the function when plotted on graph paper or the equation of the function. In the given question, we are given an equation of circle to plot on the graph that can be plotted easily once we get to know the center of the circle and the radius.
Latest Vedantu courses for you
Grade 7 | CBSE | SCHOOL | English
Vedantu 7 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
Explain why it is said like that Mock drill is use class 11 social science CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

What is the chemical name and formula of sindoor class 11 chemistry CBSE

How much is 23 kg in pounds class 11 chemistry CBSE

How do I get the molar mass of urea class 11 chemistry CBSE

What is the molecular weight of NaOH class 11 chemistry CBSE




