
Draw an angle of measure
Answer
525.6k+ views
Hint: To draw an angle of
Complete step-by-step answer:
First we will draw the angle of
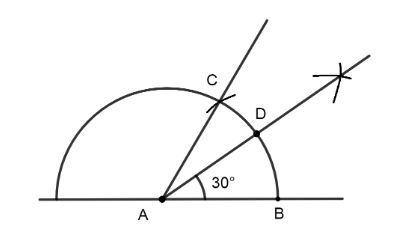
Step I: We will first draw a line segment AB of any length and then we will extend it on both sides.
Step II: Now, we will draw a semicircle of the radius = AB on this line.
Step III: Now, with the same radius, we will cut an arc on the semicircle drawn. The intersection point is named C. The angle CAB will have measure of
Step IV: From C, we will cut an arc of equal radius and from B, we will cut the arc on the arc which is already cut from C.
Step V: Now, we will join the point A to the arcs. The point at which this line intersects the line is named D. The angle
Now, we will draw the angle bisector of
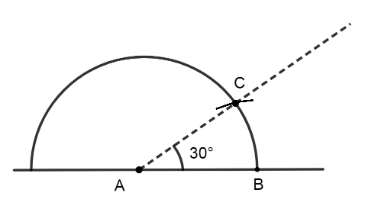
Now from B and C, we will cut arcs of the radius same as the radius of the semicircle, and then we will join the intersection point to A. The point at which the line intersects semicircle is named D. The measure of angle
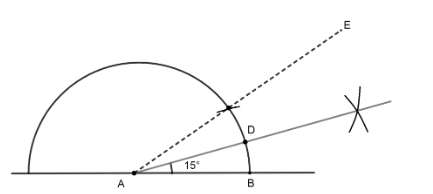
Note: To check whether the angle bisector drawn by us is correct or not, we will take radius equal to DB and then cut the arc on line AE from point D. If this arc lies on the semicircle then the angle bisector drawn by us is correct.
Complete step-by-step answer:
First we will draw the angle of

Step I: We will first draw a line segment AB of any length and then we will extend it on both sides.
Step II: Now, we will draw a semicircle of the radius = AB on this line.
Step III: Now, with the same radius, we will cut an arc on the semicircle drawn. The intersection point is named C. The angle CAB will have measure of
Step IV: From C, we will cut an arc of equal radius and from B, we will cut the arc on the arc which is already cut from C.
Step V: Now, we will join the point A to the arcs. The point at which this line intersects the line is named D. The angle
Now, we will draw the angle bisector of

Now from B and C, we will cut arcs of the radius same as the radius of the semicircle, and then we will join the intersection point to A. The point at which the line intersects semicircle is named D. The measure of angle

Note: To check whether the angle bisector drawn by us is correct or not, we will take radius equal to DB and then cut the arc on line AE from point D. If this arc lies on the semicircle then the angle bisector drawn by us is correct.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

How many ounces are in 500 mL class 8 maths CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE

Name the states through which the Tropic of Cancer class 8 social science CBSE

Explain land use pattern in India and why has the land class 8 social science CBSE

When people say No pun intended what does that mea class 8 english CBSE





