
What is the difference between medians, perpendiculars bisectors, and altitudes?
Answer
441k+ views
Hint: With reference to a triangle, the term median, perpendicular bisector and altitude seems very similar however they are different terms with different purpose. They all go to an opposite vertex. Both median and altitude start from one of the three vertices. Their differences are that the perpendicular bisector must be ninety degrees, or it'll be a median. The perpendicular line taken from the triangle's vertex to the opposite side is called the altitude.
Complete step-by-step answer:
Let us understand the difference between them:
Median: A median of a triangle is a line segment joining a vertex to the midpoint of the opposite side, thus bisecting that side. Every triangle has exactly three medians, one from each vertex, and they all intersect each other at the triangle's centroid.
Let us understand with the help of a diagram as follows:
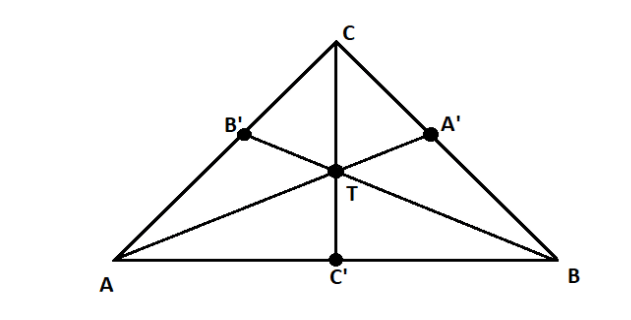
In the given figure of
Perpendicular Bisector: Perpendicular line or segment is a perpendicular line that passes through the midpoint for the side of the triangle is called perpendicular bisector.
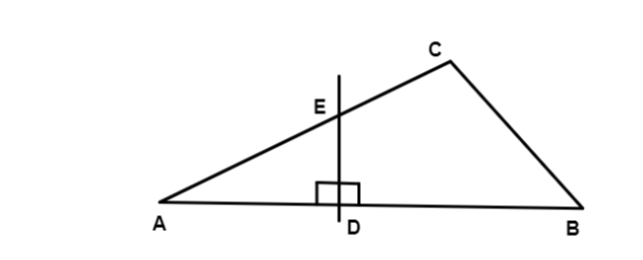
In above
Altitude: It is a line that extends from one vertex of a triangle perpendicular to the opposite side which is the base of the triangle. It is also known as the height of the triangle.
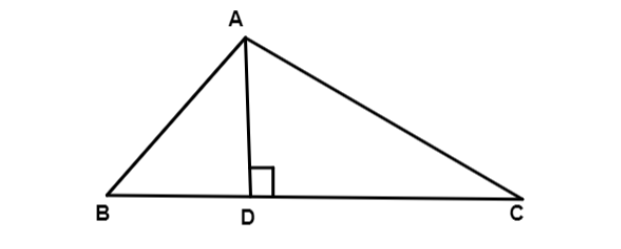
In above
Note: In the case of isosceles and equilateral triangles, a median bisects any angle at a vertex whose two adjacent sides are equal in length.
Use scale, rounder and compass to draw the medians, bisectors and altitude.
Every perpendicular bisector is a median but not every median is a perpendicular bisector.
Altitude may or may not be a perpendicular bisector of a triangle.
Complete step-by-step answer:
Let us understand the difference between them:
Median: A median of a triangle is a line segment joining a vertex to the midpoint of the opposite side, thus bisecting that side. Every triangle has exactly three medians, one from each vertex, and they all intersect each other at the triangle's centroid.
Let us understand with the help of a diagram as follows:

In the given figure of
Perpendicular Bisector: Perpendicular line or segment is a perpendicular line that passes through the midpoint for the side of the triangle is called perpendicular bisector.

In above
Altitude: It is a line that extends from one vertex of a triangle perpendicular to the opposite side which is the base of the triangle. It is also known as the height of the triangle.

In above
Note: In the case of isosceles and equilateral triangles, a median bisects any angle at a vertex whose two adjacent sides are equal in length.
Use scale, rounder and compass to draw the medians, bisectors and altitude.
Every perpendicular bisector is a median but not every median is a perpendicular bisector.
Altitude may or may not be a perpendicular bisector of a triangle.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
In Indian rupees 1 trillion is equal to how many c class 8 maths CBSE

How many ounces are in 500 mL class 8 maths CBSE

Name the states through which the Tropic of Cancer class 8 social science CBSE

How many ten lakhs are in one crore-class-8-maths-CBSE

What is the value of e infty class 8 maths CBSE

Explain land use pattern in India and why has the land class 8 social science CBSE




