
Diagonal of a rectangle are equal and perpendicular. Is this statement true? Give reason for your answer?
Answer
486k+ views
1 likes
Hint: In this question, we need to check if the diagonal of a rectangle are equal and perpendicular. For equality we will use the Pythagoras theorem according to which
Complete step by step answer:
Here we have to check if diagonals of a rectangle are equal and perpendicular to each other. Let us first draw a rectangle and label it.
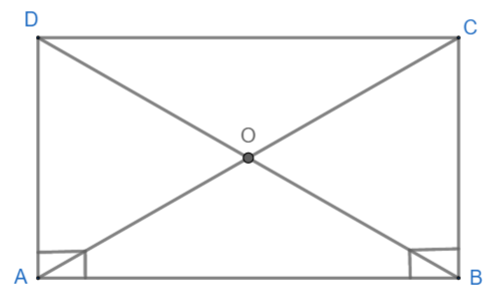
Let us suppose that, we have a rectangle ABCD with diagonals AC and BD. So we first need to prove that AC = BD.
As we know, in a rectangle adjacent sides are perpendicular to each other. Therefore,
So from the figure, we can say that,
Pythagoras theorem is given as
From
Also
Now we know that the opposite sides in a rectangle are equal therefore, AD = BC. Putting it in equation (2) we get,
From (1) and (3) we can see that
Taking square root on both sides we get, AC = BD.
Hence diagonal of a rectangle are equal to each other.
Now for checking if they are perpendicular. Let us assume that
Then in triangle
OB = OB
AO = OC (diagonals of a rectangle bisect each other).
So triangles
But AB = BC only in the case of square whereas we have a rectangle whose adjacent sides may not be equal. These constraints our assumption.
Therefore,
Hence diagonal of a rectangle need not be perpendicular to each other.
So the given statement is not true.
Note:
Students should note that a square is a type of rectangle that has diagonals that are perpendicular to each other. So the statement is not entirely false but it is just not applicable to all rectangles. Try to keep in mind the properties of the quadrilateral.
Complete step by step answer:
Here we have to check if diagonals of a rectangle are equal and perpendicular to each other. Let us first draw a rectangle and label it.

Let us suppose that, we have a rectangle ABCD with diagonals AC and BD. So we first need to prove that AC = BD.
As we know, in a rectangle adjacent sides are perpendicular to each other. Therefore,
So from the figure, we can say that,
Pythagoras theorem is given as
From
Also
Now we know that the opposite sides in a rectangle are equal therefore, AD = BC. Putting it in equation (2) we get,
From (1) and (3) we can see that
Taking square root on both sides we get, AC = BD.
Hence diagonal of a rectangle are equal to each other.
Now for checking if they are perpendicular. Let us assume that
Then in triangle
OB = OB
AO = OC (diagonals of a rectangle bisect each other).
So triangles
But AB = BC only in the case of square whereas we have a rectangle whose adjacent sides may not be equal. These constraints our assumption.
Therefore,
Hence diagonal of a rectangle need not be perpendicular to each other.
So the given statement is not true.
Note:
Students should note that a square is a type of rectangle that has diagonals that are perpendicular to each other. So the statement is not entirely false but it is just not applicable to all rectangles. Try to keep in mind the properties of the quadrilateral.
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




