
How do you convert complex numbers from standard form to polar form and vice versa?
Answer
468.3k+ views
Hint: Assume any complex number of the standard form
Complete step by step answer:
Here, we have been asked to convert a complex number from its standard form to the polar form and from its polar form to the standard form. So, if we will get the relation between the two forms then we can easily convert it from one form to the other. Here, we will try to convert the standard form to the polar form.
Now, we know that the standard form of a complex number is
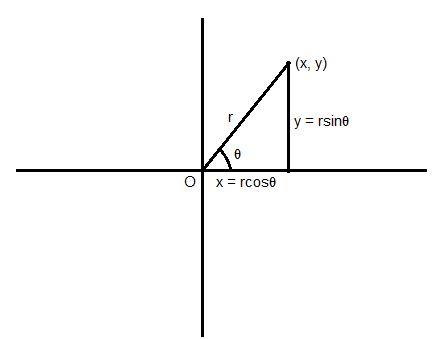
So, the magnitude of this position vector is given as
Now, we have to calculate the value of
Therefore, the complex number in polar form becomes,
Hence, the above relation is our answer.
Note:
Now, if you are provided with the complex number in polar form
Complete step by step answer:
Here, we have been asked to convert a complex number from its standard form to the polar form and from its polar form to the standard form. So, if we will get the relation between the two forms then we can easily convert it from one form to the other. Here, we will try to convert the standard form to the polar form.
Now, we know that the standard form of a complex number is

So, the magnitude of this position vector is given as
Now, we have to calculate the value of
Therefore, the complex number in polar form becomes,
Hence, the above relation is our answer.
Note:
Now, if you are provided with the complex number in polar form
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 10 Computer Science: Engaging Questions & Answers for Success

Master Class 10 Maths: Engaging Questions & Answers for Success

Master Class 10 English: Engaging Questions & Answers for Success

Master Class 10 General Knowledge: Engaging Questions & Answers for Success

Master Class 10 Science: Engaging Questions & Answers for Success

Master Class 10 Social Science: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




