
Construct a triangle of sides 5 cm, 6cm and 7cm and then a triangle similar to it whose sides are
Answer
504.6k+ views
Hint: Here first we will construct the triangle with sides 5 cm, 6cm, and 7cm and then we will use the given fraction of the side to construct a similar triangle.
Complete step by step answer:
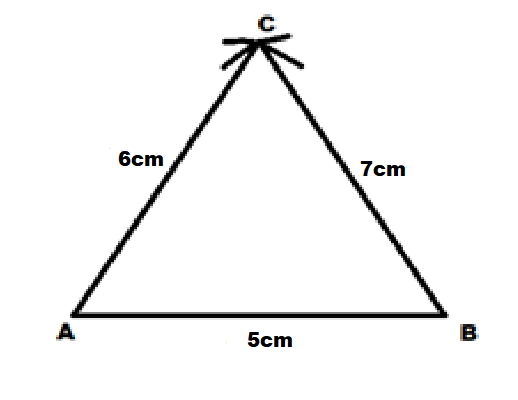
Let us first draw the triangle with sides 5 cm, 6cm and 7cm.
- First, we will draw the base AB of the triangle of length 5cm.
- Then A as the center we will mark an arc with the help of a compass at a distance of 6cm.
- Similarly, now with B as the center, we will mark an arc with the help of a compass at a distance of 7cm.
- Now finally we will mark the point so obtained as C and then join the points A and C and A and B.
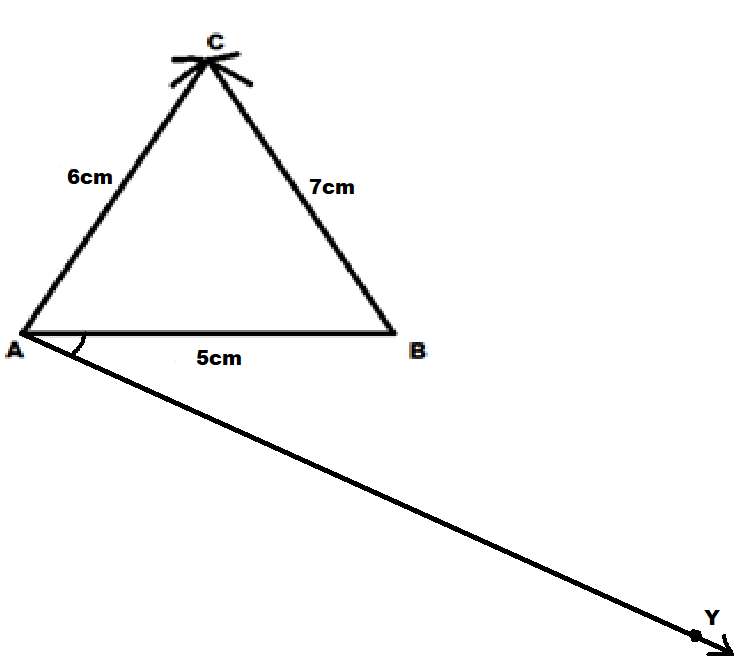
Now we will construct a similar triangle of ABC whose sides are
We will first construct a ray AY such that it makes an acute angle with line AB on the opposite side of vertex C.
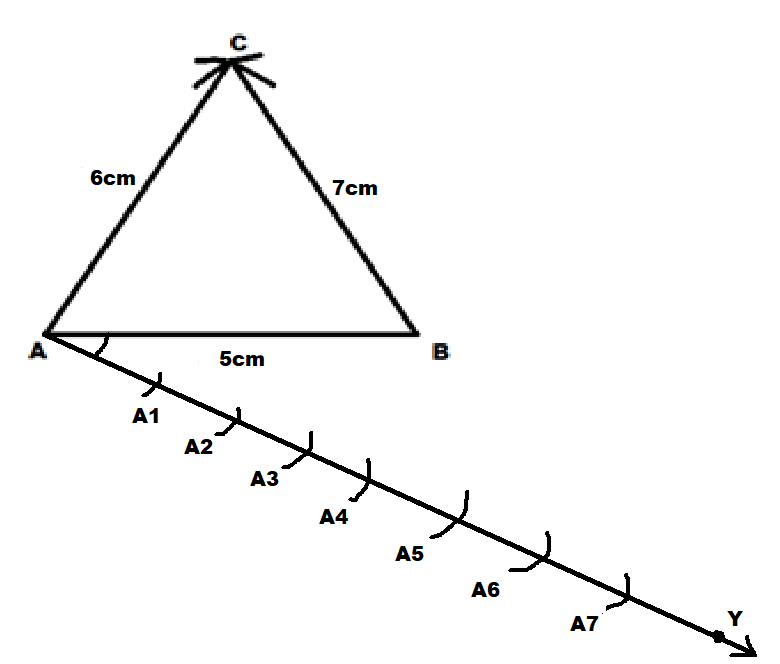
Now we will mark seven points (as 7 is greater than 5 in the fraction
Now we will join
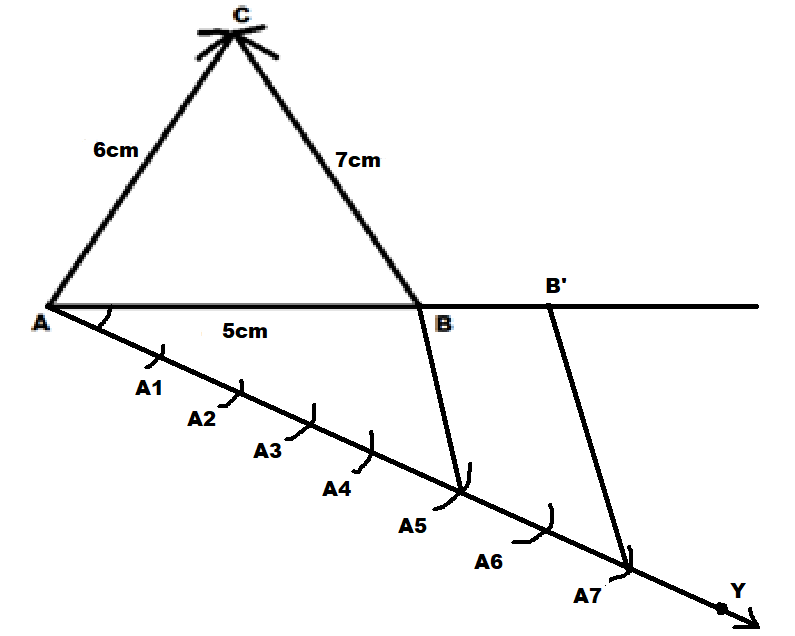
Now we will finally draw a line from
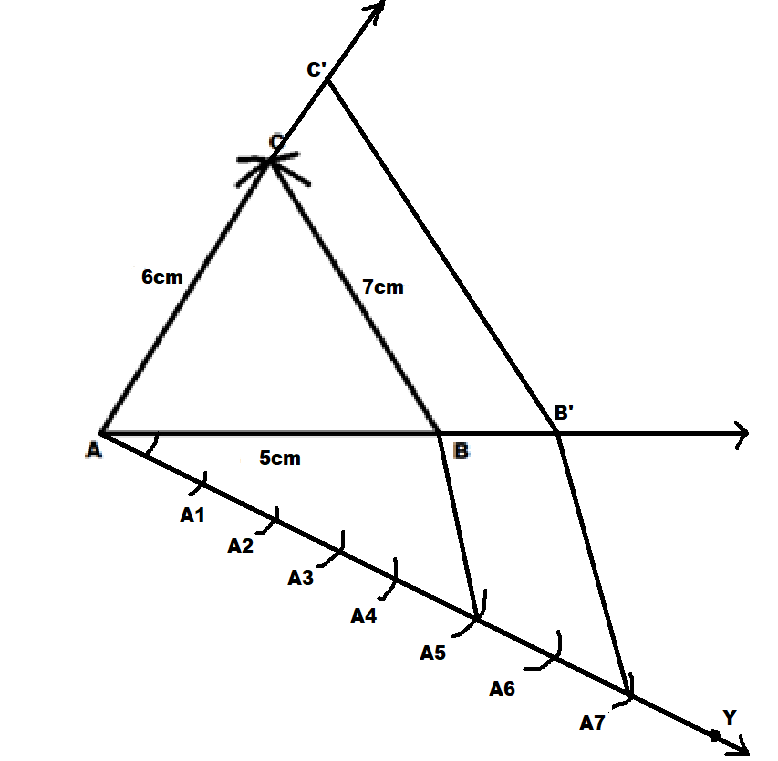
Now the new triangle so formed i.e.
Note:
- Students should use compass and scale for perfect measurement and arcs an accurate distance to get the desired triangles.
- Also, we have drawn three arcs on the ray AY because we had to construct a similar triangle such that its sides are
Complete step by step answer:
Let us first draw the triangle with sides 5 cm, 6cm and 7cm.
- First, we will draw the base AB of the triangle of length 5cm.
- Then A as the center we will mark an arc with the help of a compass at a distance of 6cm.
- Similarly, now with B as the center, we will mark an arc with the help of a compass at a distance of 7cm.
- Now finally we will mark the point so obtained as C and then join the points A and C and A and B.

Now we will construct a similar triangle of ABC whose sides are
We will first construct a ray AY such that it makes an acute angle with line AB on the opposite side of vertex C.

Now we will mark seven points (as 7 is greater than 5 in the fraction

Now we will join

Now we will finally draw a line from

Now the new triangle so formed i.e.
Note:
- Students should use compass and scale for perfect measurement and arcs an accurate distance to get the desired triangles.
- Also, we have drawn three arcs on the ray AY because we had to construct a similar triangle such that its sides are
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Difference between mass and weight class 10 physics CBSE

Leap year has days A 365 B 366 C 367 D 368 class 10 maths CBSE

What is Whales collective noun class 10 english CBSE

Write 10 lines on the usefulness of microorganisms class 10 biology CBSE

Describe the Salient Features of Indian Economy




