
How do you calculate the surface area of the lateral faces of a regular hexagonal pyramid that has a slant height of 35 cm and a base side length of 4 cm?
Answer
482.4k+ views
1 likes
Hint: We recall the shape of a regular hexagonal pyramid which has lateral faces in congruent triangular shape. We find the area of one triangular base and multiply 6 to get the answer. We find the area of one triangle as
Complete step by step answer:
We know that a Pyramid is a polyhedron formed by connecting a polygonal base and a point, called the apex. Each base edge and apex form a triangle, called a lateral face. A right pyramid has its apex directly above the centroid of its base. A pyramid that has a regular polygon base is called a regular pyramid. A regular hexagonal pyramid has its base as a regular hexagon. We draw the rough figure of it below with O as the apex and ABCDEF as the base of a regular hexagonal base.
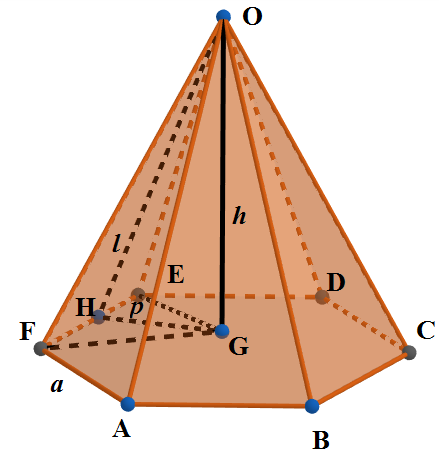
The altitude dropped from apex on the hexagon is called height of the pyramid
The surfaces excluding the base are called lateral surfaces and in a regular pyramid they are congruent. We have 6 lateral faces in a regular hexagonal pyramid which are in triangular shape. Since they are congruent they will be of equal area. So the surface area of the lateral faces will be 6 times the area of one triangular face.
Let us observe the triangle OEH. We know that the area of one triangle is
The regular hexagonal pyramid has a slant height of 35 cm and a base side length of 4 cm. So we have
We multiply 6 and find the surface area of all the lateral faces (LSA) as
Note: We note that the altitude from the centre of the hexagon on a side (here
Complete step by step answer:
We know that a Pyramid is a polyhedron formed by connecting a polygonal base and a point, called the apex. Each base edge and apex form a triangle, called a lateral face. A right pyramid has its apex directly above the centroid of its base. A pyramid that has a regular polygon base is called a regular pyramid. A regular hexagonal pyramid has its base as a regular hexagon. We draw the rough figure of it below with O as the apex and ABCDEF as the base of a regular hexagonal base.

The altitude dropped from apex on the hexagon is called height of the pyramid
The surfaces excluding the base are called lateral surfaces and in a regular pyramid they are congruent. We have 6 lateral faces in a regular hexagonal pyramid which are in triangular shape. Since they are congruent they will be of equal area. So the surface area of the lateral faces will be 6 times the area of one triangular face.
Let us observe the triangle OEH. We know that the area of one triangle is
The regular hexagonal pyramid has a slant height of 35 cm and a base side length of 4 cm. So we have
We multiply 6 and find the surface area of all the lateral faces (LSA) as
Note: We note that the altitude from the centre of the hexagon on a side (here
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which of the following districts of Rajasthan borders class 9 social science CBSE

What is 85 of 500 class 9 maths CBSE

What is the full form of pH?

Define human made resources

India has states and Union Territories A 29 7 B 28 class 9 social science CBSE

What is the importance of natural resources? Why is it necessary to conserve them?




