
An object A of mass
(A)
(B)
(C)
(D)
Answer
451.5k+ views
Hint : It is known that for an elastic collision total energy and momentum due to collision is always conserved. Use conservation of momentum to find the mass of B. The conservation of momentum states that the momentum of a system in collision is always a constant of motion.
Complete Step By Step Answer:
We have given here that, the object A of mass
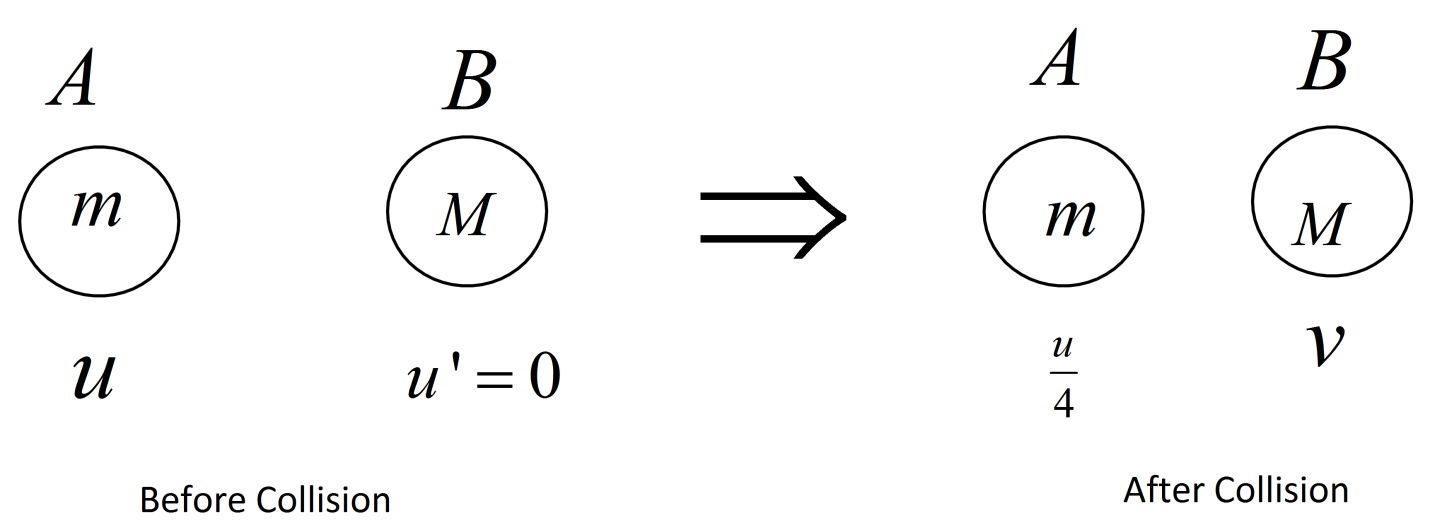
Hence, we can write,
Or,
So, we get,
Now, from conservation of momentum we know that momentum of the collision is conserved. Hence, momentum after collision is equal to the momentum before collision
So, we can write,
So, putting the value of the final velocity of the object B,
Simplifying further we get,
Hence,
Hence, the mass of the object B will be,
Hence, option (B ) is correct.
Note :
Conservation of mechanical energy states that the total mechanical energy of a system due to an elastic collision is always constant. If the potential energy of the system is taken to be constant then we can write,
Complete Step By Step Answer:
We have given here that, the object A of mass
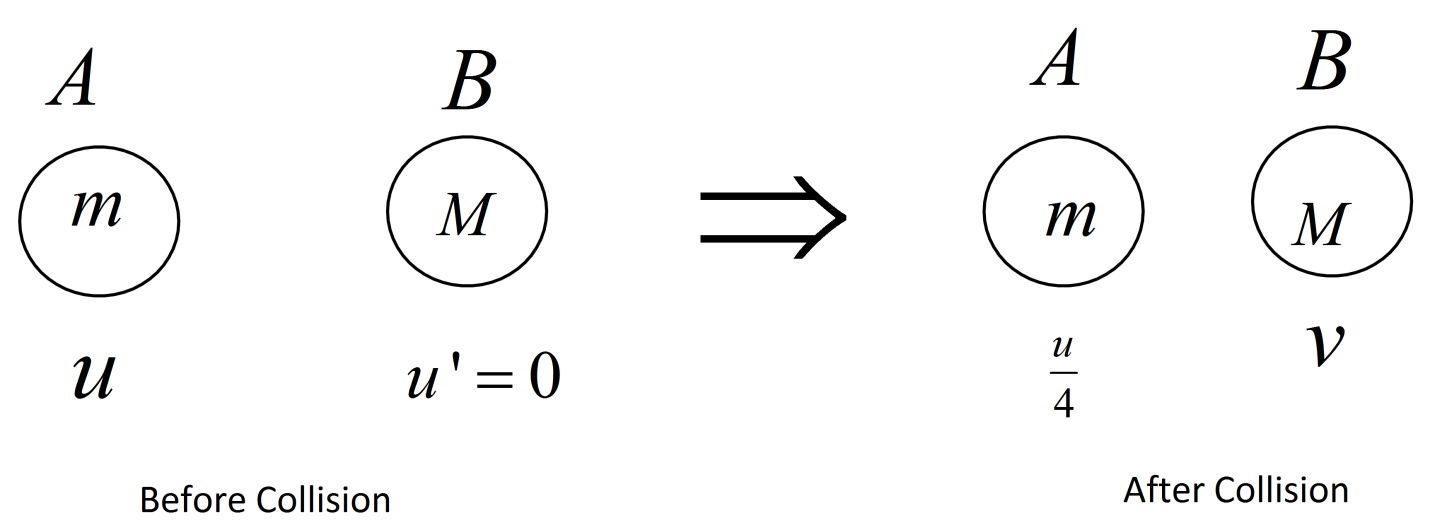
Hence, we can write,
Or,
So, we get,
Now, from conservation of momentum we know that momentum of the collision is conserved. Hence, momentum after collision is equal to the momentum before collision
So, we can write,
So, putting the value of the final velocity of the object B,
Simplifying further we get,
Hence,
Hence, the mass of the object B will be,
Hence, option (B ) is correct.
Note :
Conservation of mechanical energy states that the total mechanical energy of a system due to an elastic collision is always constant. If the potential energy of the system is taken to be constant then we can write,
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
Explain why it is said like that Mock drill is use class 11 social science CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE




