Question

$ABC$ is a triangle right angled at $C$. A line through the midpoint $M$ of hypotenuse $AB$ and parallel to $BC$ intersects $AC$ and $D$. Show that
(i) $D$ is the midpoint of $AC$
(ii) $MB \bot \;AC$
(iii) $CM = MA = \dfrac{1}{2}AB$

Answer 1

Hint: In the solution we will use the similar triangle properties. The properties may contain the Angle Angle Angle (AAA), Side Angle Side (SAS), Side Side Side (SSS) and Right angle Hypotenuse Side (RHS) properties.

Complete Step-by-step Solution
Given: The triangle $\Delta ABC$ is a right-angled triangle having angle $\angle C = 90^\circ $ and $M$ is the midpoint of $AB$.

The following is the schematic diagram of triangle $\Delta ABC$.
                        

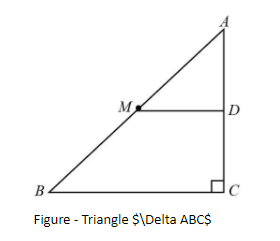
(i)We know that, in the right-angled triangle $\Delta ABC$, $M$ is the midpoint of line $AB$ and $MD\parallel BC$. So, according to the mid-point theorem it can be said that $D$ is the midpoint of $AC$.

Hence, it is proved that $D$ is the midpoint of $AC$.

(ii)Since, we can observe from the diagram in the right-angled triangle $\Delta ABC$, the line MD is parallel to BC that is $MD\;\parallel \;BC$ and $AC$ is transversal.

Also, we know that the interior angles on the same side of transversal AC are supplementary that is,
$\angle MDC + \angle BCD = 180^\circ $

On putting $90^\circ $ for $\angle BCD$ in the above relation we get the value of angle $MDC$.
$\begin{array}{c}
\angle MDC + 90^\circ = 180^\circ \\
\angle MDC = 90^\circ
\end{array}$

Therefore, $MB \bot \;AC$ because the angle $\angle MDC = 90^\circ $.

(iii)Now, according to Right-angle Hypotenuse Side (RHS) similar triangle properties in $\Delta AMD$ and $\Delta CMD$.
$\begin{array}{c}
AD = CD\\
\angle ADM = \angle CDM\\
DM = DM
\end{array}$
So, the triangles $\Delta AMD \cong \Delta CMD$ are congruent through SAS congruence rule.
Now, we know by the rule of CPCT,
$AM = CM$……(1)
Since, we know that $AM = \dfrac{1}{2}AB$ because it is given that M is the midpoint of AB.
$AM = \dfrac{1}{2}AB$….(2)
On equating the equations (1) and (2), we get the value as,
$CM = AM = \dfrac{1}{2}AB$
Therefore, it is proved that $CM = AM = \dfrac{1}{2}AB$.

Note: Make sure to use a rationalization method when you will see this type of questions. The tricky part is comparing the powers of the equations.