Question

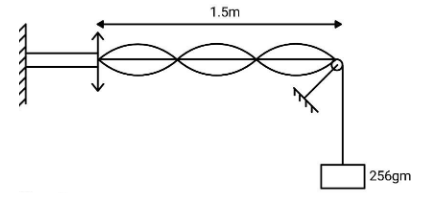
A string is stretched between a pulley and a wave generator consisting of a plate vibrating up and down with small amplitude and frequency $120Hz$. The standing wave pattern has 4 nodes as shown. What should be the load (in gm) if we want a standing wave with 5 nodes?

Answer 1

Hint: Here, you are given a setup of a string, a pulley, a wave generator and a block of mass $256gm$. This question is based on finding quantities from one case and then using those to find unknown quantities of other cases. In order to solve this question, firstly what you need to do is obtain an expression for frequency in terms of length of the string and velocity of the wave, the velocity of the wave can be found out in terms of tension in the string and mass density of the string. Also, it is given that there are 4 nodes and 2 of them are at the ends, so the ends can be considered as fixed ends.

Complete step by step answer:
Let us first see the frequency of standing waves on a string with both ends fixed. As you know that the frequency is given as $f={\displaystyle \frac{v}{\lambda }}$, where $v$ is the velocity of the wave and $\lambda$ is the wavelength of the wave. As you can see that since there are nodes at both the ends, there will be a number of loops with length equal to the wavelength of the wave, that is ${\displaystyle \frac{\lambda }{2}}$.

Let us stop at this argument and discuss standing waves. Consider a wave which is travelling from the generator to the fixed end, the wave gets reflected at the fixed end, gets inverted (according to Newton’s third law of motion) and travels back to the generator. The generator is also acting as a fixed end and therefore it again gets reflected and again is inverted. You can imagine that our wave will interfere constructively with the newly generated wave. Now, we will come back to our equation of frequency. If we have the length of string as an integral multiple of ${\displaystyle \frac{\lambda }{2}}$, this situation will always be satisfied. So, $l={\displaystyle \frac{n\lambda }{2}}$ and we have frequency as $f={\displaystyle \frac{nv}{2l}}$.

Velocity of a wave in string is given by $v=\sqrt{{\displaystyle \frac{T}{\mu }}}$, where $T$ is tension in the string and $\mu$ is mass per unit length, that is linear mass density.
First case: 4 nodes, mass $=256gm=0.256kg$ $\to$ weight $=mg=\left(0.256\right)\left(10\right)=2.56N$ and tension will be equal to weight as the block is in equilibrium, $\therefore T=2.56N$, frequency $f=120Hz$, length $l=1.5m$. The only unknown is $\mu$.
$$\begin{gathered} f={\displaystyle \frac{n}{2l}}\sqrt{{\displaystyle \frac{T}{\mu }}} \\ \Rightarrow 120={\displaystyle \frac{3}{\left(2\right)\left(1.5\right)}}\sqrt{{\displaystyle \frac{2.56}{\mu }}} \\ \Rightarrow {\left(120\right)}^2={\displaystyle \frac{2.56}{\mu }} \\ \Rightarrow \mu ={\displaystyle \frac{2.56}{{\left(120\right)}^2}} \end{gathered}$$
Second case: 5 nodes (4 loops), let the mass be $m$, so the tension will be $T=mg$, frequency $f=120Hz$, length $l=1.5m$ and $\mu ={\displaystyle \frac{2.56}{{\left(120\right)}^2}}$.
$$\begin{gathered} f={\displaystyle \frac{n}{2l}}\sqrt{{\displaystyle \frac{T}{\mu }}} \\ \Rightarrow 120={\displaystyle \frac{4}{\left(2\right)\left(1.5\right)}}\sqrt{{\displaystyle \frac{mg}{{\displaystyle \frac{2.56}{{\left(120\right)}^2}}}}} \\ \Rightarrow m=0.144kg \\ \therefore m=144gm \end{gathered}$$
Therefore, $144gm$ should be the load if we want a standing wave with 5 nodes.

Note: Here, we have discussed in detail about the frequency of the waves that should be possessed by the string in order to achieve constructive interference, so you should keep in mind the argument we made. Also, for both ends fixed we have length is integral multiple of half of wavelength and for only one end fixed, we have length is odd integral multiple of one fourth of wavelength.