Question

A solid is in the shape of a cone standing on a hemisphere with both their radii being equal to 1 cm and height of the cone is equal to its radius. Find the volume of the solid in terms of $\pi $.

Answer 1

Hint: We will apply the formula of volume of a cone given by $\dfrac{1}{3}\pi {{r}^{2}}h$ and volume of hemisphere given by $\dfrac{2}{3}\pi {{r}^{3}}$ where the radius of the cone and hemisphere both are equal whereas h is the height of the cone. We will apply this formula in order to solve the question further for the volume of the solid.

Complete step-by-step answer:
According to the question the diagram of the solid is given by the following figure.

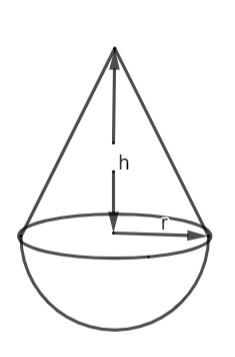
Clearly, we can see that the total volume of the solid is made by the cone and a hemisphere. Therefore, the volume of the whole solid will be the volume of the cone and the volume of the sphere together. Now, we will apply the formula for the volume of the cone which is given by $\dfrac{1}{3}\pi {{r}^{2}}h$. Here r is the radius of the cone and h is the height of the cone. Since, we are given the question that the radius of the cone is equal to the height of the cone.
$\begin{align}
  & \Rightarrow vol.\,\,of\,\,cone=\dfrac{1}{3}\pi {{r}^{2}}h \\
 & \Rightarrow vol.\,\,of\,\,cone=\dfrac{1}{3}\pi {{r}^{2}}r \\
 & \Rightarrow vol.\,\,of\,\,cone=\dfrac{1}{3}\pi {{r}^{3}} \\
\end{align}$
As r = 1 cm.
$\begin{align}
  & \Rightarrow vol.\,\,of\,\,cone=\dfrac{1}{3}\pi {{\left( 1 \right)}^{3}}c{{m}^{3}} \\
 & \Rightarrow vol.\,\,of\,\,cone=\dfrac{1}{3}\pi c{{m}^{3}} \\
\end{align}$
Now, we will find the volume of the hemisphere by the formula given by $\dfrac{2}{3}\pi {{r}^{3}}$ where r is the radius of the hemisphere.
$\Rightarrow vol.\,\,of\,\,hemisphere\,=\,\dfrac{2}{3}\pi {{r}^{3}}$
Since, the radius of cone, hemisphere and solid is the same. Thus, we can write that the radius of the hemisphere is equal to 1 cm.
$\begin{align}
  & vol.\,\,of\,\,hemisphere\,=\,\dfrac{2}{3}\pi {{r}^{3}} \\
 & \Rightarrow vol.\,\,of\,\,hemisphere\,=\,\dfrac{2}{3}\pi {{\left( 1 \right)}^{3}}c{{m}^{3}} \\
 & \Rightarrow vol.\,\,of\,\,hemisphere\,=\,\dfrac{2}{3}\pi c{{m}^{3}} \\
\end{align}$
Now, we will add the volume of the cone with the volume of the hemisphere to find the volume of the solid. Therefore, the volume of solid = volume of cone + volume of hemisphere.
$\begin{align}
  & \Rightarrow vol.\,\,of\,\,solid\,=\,\dfrac{1}{3}\pi c{{m}^{3}}+\dfrac{2}{3}\pi c{{m}^{3}} \\
 & \Rightarrow vol.\,\,of\,\,solid\,=\,\dfrac{3}{3}\pi c{{m}^{3}} \\
 & \Rightarrow vol.\,\,of\,\,solid\,=\,\pi c{{m}^{3}} \\
\end{align}$
Hence, the volume of the solid is $\pi c{{m}^{3}}$ which is in terms of $\pi $.
Note: In making the diagram of the solid one can draw the cone below the hemisphere but it will only be right if there are no specifications of the solid in the question. But in the question it is clearly saying that the cone is standing on the hemisphere. So, there is no other option of drawing the solid. It should be as in the solution drawn by. In writing the volume one can consider radius r = 1 instead of 1 cm, which results into the volume without any unit. This will become the wrong answer.