
A solid is composed of a cylinder with hemispherical ends. If the whole length of solid is 108 cm and the diameter of the hemispherical ends is 36 cm, find the cost of polishing its surface at the rate of 7 paise per sq. cm.
Answer
537k+ views
1 likes
Hint: Determine the length of the cylinder and then the curved surface area of the cylinder, that is,
Complete step-by-step answer:
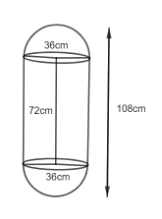
We need to find the cost of polishing the solid composed of a cylinder with hemispherical ends.
Hence, we need to find the total surface area of the solid. It is equal to the sum of the curved surface area of the cylinder and surface area of the two hemispheres.
The total length of the solid is the sum of the length of the cylinder and the diameter of the hemispheres.
Then, the length of the cylinder part alone is given by:
The radius of the solid is given by,
We know that the curved surface area of a cylinder with radius r and length h is given as follows:
The curved surface area of the cylinder with radius 18 cm and length 72 cm is given by equation (1) as follows:
We, now, find the surface area of the hemispherical ends.
The surface area of a hemisphere is given by:
The surface area of two hemispherical ends of radius 18 cm is given as follows:
The total surface area of the solid is then the sum of equation (3) and equation (4).
The cost of polishing is 7 paise per sq. cm.
Hence, the total cost of painting is given as follows:
Total cost =
Total cost = 85536 paise
Total cost = Rs. 855.36
Hence, the total cost of painting the solid is Rs. 855.36.
Note: Note that the total length of the solid is 108 cm and not the length of the cylindrical part alone. Hence, calculate the length of the cylindrical part first before calculating its curved surface area.
Complete step-by-step answer:

We need to find the cost of polishing the solid composed of a cylinder with hemispherical ends.
Hence, we need to find the total surface area of the solid. It is equal to the sum of the curved surface area of the cylinder and surface area of the two hemispheres.
The total length of the solid is the sum of the length of the cylinder and the diameter of the hemispheres.
Then, the length of the cylinder part alone is given by:
The radius of the solid is given by,
We know that the curved surface area of a cylinder with radius r and length h is given as follows:
The curved surface area of the cylinder with radius 18 cm and length 72 cm is given by equation (1) as follows:
We, now, find the surface area of the hemispherical ends.
The surface area of a hemisphere is given by:
The surface area of two hemispherical ends of radius 18 cm is given as follows:
The total surface area of the solid is then the sum of equation (3) and equation (4).
The cost of polishing is 7 paise per sq. cm.
Hence, the total cost of painting is given as follows:
Total cost =
Total cost = 85536 paise
Total cost = Rs. 855.36
Hence, the total cost of painting the solid is Rs. 855.36.
Note: Note that the total length of the solid is 108 cm and not the length of the cylindrical part alone. Hence, calculate the length of the cylindrical part first before calculating its curved surface area.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Which tributary of Indus originates from Himachal Pradesh class 10 social science CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Distinguish between ordinary light and laser light class 10 physics CBSE




