
A rectangular container has a base of length 12cm and width 9cm. A cube of edge 6cm is placed in the container and then sufficient water is filled into it so that the cube is just submerged. Find the fall in the level of water, in the container, when the cube is removed.
A. 2cm
B. 1cm
C. 4cm
D. 3cm
Answer
498.6k+ views
Hint:
Assume the height of water filled to just submerge the cube to be h and the drop in height of water after removal of the cube be x cm.
So, the new height of water in the container will be
In the whole process of removing the cube and decreasing the height of water, the volume of water filled up in the container before removing the cube and after removal will remain the same. Find the two volumes and equate them for finding x.
Formula used:
Volume of a cuboid is given by:
Where, l, b and h are length, breadth and height of the cuboid respectively.
Volume of a cube is given by:
Complete step by step solution:
It is given that the rectangular container has a base of dimensions
Let us assume that, the height of water filled just to submerge the cube of side 6cm be h.
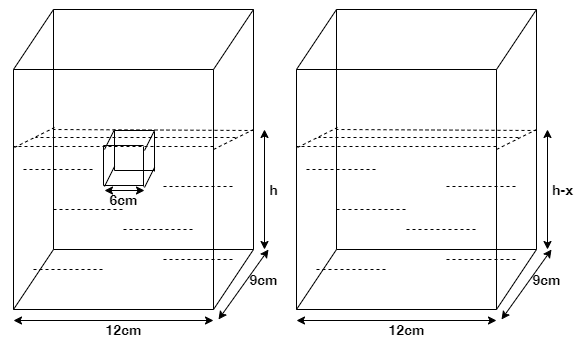
Now, let us assume the height of water dropped after removing the cube is
So, the new height of water will be
In the whole process of removing the cube and decreasing the height of water, the volume of water filled up in the container before removing the cube and after removal will remain the same.
So, first find the volume of water before removal of the cube of side 6cm.
It will be given by subtracting the volume of the cube of side 6cm from the volume of the container with rectangular base of dimensions
Volume of water (before removal of cube) = volume of container up to height h - volume of cube
Volume of water (after removal of cube) = volume of container up to height
As told,
Volume of water (before removal of cube) = Volume of water (after removal of cube)
Cancelling 108h from both sides, we get:
Hence, the drop in the height of water will be 2 cm.
Note:
This question can also be approached in the given manner.
Assuming the height of water dropped to be x cm.
The removal of the cube from the container is similar to the removal of volume of water of cross-section
Therefore,
Assume the height of water filled to just submerge the cube to be h and the drop in height of water after removal of the cube be x cm.
So, the new height of water in the container will be
In the whole process of removing the cube and decreasing the height of water, the volume of water filled up in the container before removing the cube and after removal will remain the same. Find the two volumes and equate them for finding x.
Formula used:
Volume of a cuboid is given by:
Where, l, b and h are length, breadth and height of the cuboid respectively.
Volume of a cube is given by:
Complete step by step solution:
It is given that the rectangular container has a base of dimensions
Let us assume that, the height of water filled just to submerge the cube of side 6cm be h.

Now, let us assume the height of water dropped after removing the cube is
So, the new height of water will be
In the whole process of removing the cube and decreasing the height of water, the volume of water filled up in the container before removing the cube and after removal will remain the same.
So, first find the volume of water before removal of the cube of side 6cm.
It will be given by subtracting the volume of the cube of side 6cm from the volume of the container with rectangular base of dimensions
Volume of water (before removal of cube) = volume of container up to height h - volume of cube
Volume of water (after removal of cube) = volume of container up to height
As told,
Volume of water (before removal of cube) = Volume of water (after removal of cube)
Cancelling 108h from both sides, we get:
Hence, the drop in the height of water will be 2 cm.
Note:
This question can also be approached in the given manner.
Assuming the height of water dropped to be x cm.
The removal of the cube from the container is similar to the removal of volume of water of cross-section
Therefore,
Recently Updated Pages
Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Chemistry: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




