
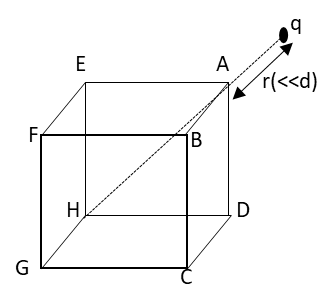
A point charge
A.
B.
C.
D.

Answer
491.4k+ views
Hint: Use the formula for flux of electric field passing through a surface. Construct a Gaussian surface for the surface ABCD of the cube. Determine the total flux through this Gaussian surface and from these calculations determine the flux passing though the surface ABCD and its direction.
Formula used:
The magnetic flux
Here,
Complete step by step answer:
We have given that a point charge
The length of each side of the cube is
We have asked to determine the total flux of electric field through the surface ABCD of the cube.
But we know that the electric flux is always through a Gaussian surface i.e. a closed surface and ABCD is not a close surface.
Hence, let us draw a Gaussian surface including the surface ABCD of the given cube as follows:
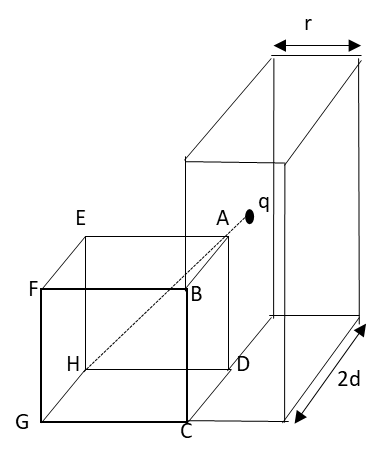
Now the above constructed surface around the surface ABCD is a Gaussian surface of sides of cube as
Since the length
Hence, there remains only two surfaces of the Gaussian surface with sides
Hence, the total flux
Also, the flux
Substitute
Substitute
The negative sign indicates that the direction of the total flux is in the negative direction.
Therefore, the magnetic flux passing through the surface ABCD is
So, the correct answer is “Option A”.
Note:
The students may think how the direction of the flux through the surface ABCD is in a negative direction. But we know that the direction of the flux due to positive charge is outward on all surfaces. Hence, the direction of flux for surface ABCD will be outwards from charge in the left hand direction which is the negative direction.
Formula used:
The magnetic flux
Here,
Complete step by step answer:
We have given that a point charge
The length of each side of the cube is
We have asked to determine the total flux of electric field through the surface ABCD of the cube.
But we know that the electric flux is always through a Gaussian surface i.e. a closed surface and ABCD is not a close surface.
Hence, let us draw a Gaussian surface including the surface ABCD of the given cube as follows:

Now the above constructed surface around the surface ABCD is a Gaussian surface of sides of cube as
Since the length
Hence, there remains only two surfaces of the Gaussian surface with sides
Hence, the total flux
Also, the flux
Substitute
Substitute
The negative sign indicates that the direction of the total flux is in the negative direction.
Therefore, the magnetic flux passing through the surface ABCD is
So, the correct answer is “Option A”.
Note:
The students may think how the direction of the flux through the surface ABCD is in a negative direction. But we know that the direction of the flux due to positive charge is outward on all surfaces. Hence, the direction of flux for surface ABCD will be outwards from charge in the left hand direction which is the negative direction.
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Why is insulin not administered orally to a diabetic class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

How do you convert from joules to electron volts class 12 physics CBSE

Define Vant Hoff factor How is it related to the degree class 12 chemistry CBSE

The first microscope was invented by A Leeuwenhoek class 12 biology CBSE




