
A constraint in an LP model becomes redundant because
A. Two iso-profit lines may be parallel to each other
B. The solution is unbounded
C. The constraint is not satisfied by the solution values
D. None of the above
Answer
453.4k+ views
Hint: We recall the definitions of iso-profit lines, unbounded solution, feasible region and redundant constraints. We show with a two-variable cost function that the feasible region doesn’t change because of redundant constraints and is not related to iso-profit lines, unbounded solutions.
Complete step-by-step solution
We know that in linear programming problems or LPP the output is the profit or cost function. The cost or profit function is the function which has to be optimized that is minimized or maximized. It is expressed in the standard from of the LPP with
We suppose that the cost has to be minimized. We define the problem constraints ( for the cost function as
The non-negative constraints are,
The redundant constraint of the constraint
Let us observe it for 2 variables
We have to maximize it. We have the non-negative constraint
We convert all the inequality constraints into equalities and plot all the lines to find a graphical solution.
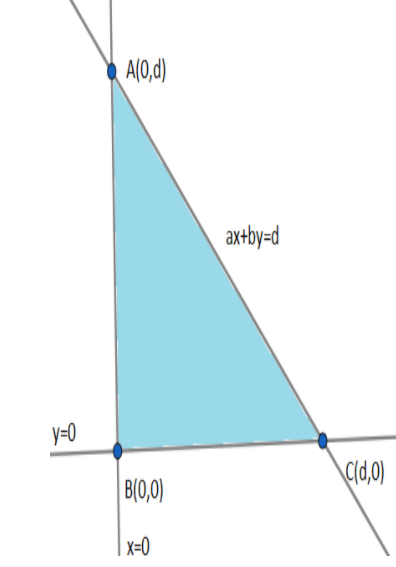
The blue shaded region of the interior triangle ABC is called feasible because all the points on it satisfy the problem constraints and non-negative constraints. Every point in the feasible region is called a feasible solution and the vertex where
If we plot the above constraint by converting to equality it will be the same line as AC and it won’t change the feasible region. Let us check the options now.
A. Iso-profit lines are the lines representing the cost function and their parallel existence is not related to the redundant constraint. So A is not correct.
B. If the feasible region is not a closed curve and each point is called an unbounded solution. It is not related to redundancy, its constraints, and B is incorrect.
C. A point outside the feasible region may not be satisfied by one or more of the constraints. It is not related to redundancy, is constrained and C is incorrect.
So the correct option is D.
Note: There are different methods to deal and remove redundant constraints in LPP for example using linear combinations in vector spaces. We also note that the feasible region is convex set which means the line segment joining two points in the region will not have any point outside it.
Complete step-by-step solution
We know that in linear programming problems or LPP the output is the profit or cost function. The cost or profit function is the function which has to be optimized that is minimized or maximized. It is expressed in the standard from of the LPP with
We suppose that the cost has to be minimized. We define the problem constraints ( for the cost function as
The non-negative constraints are,
The redundant constraint of the constraint
Let us observe it for 2 variables
We have to maximize it. We have the non-negative constraint
We convert all the inequality constraints into equalities and plot all the lines to find a graphical solution.

The blue shaded region of the interior triangle ABC is called feasible because all the points on it satisfy the problem constraints and non-negative constraints. Every point in the feasible region is called a feasible solution and the vertex where
If we plot the above constraint by converting to equality it will be the same line as AC and it won’t change the feasible region. Let us check the options now.
A. Iso-profit lines are the lines representing the cost function and their parallel existence is not related to the redundant constraint. So A is not correct.
B. If the feasible region is not a closed curve and each point is called an unbounded solution. It is not related to redundancy, its constraints, and B is incorrect.
C. A point outside the feasible region may not be satisfied by one or more of the constraints. It is not related to redundancy, is constrained and C is incorrect.
So the correct option is D.
Note: There are different methods to deal and remove redundant constraints in LPP for example using linear combinations in vector spaces. We also note that the feasible region is convex set which means the line segment joining two points in the region will not have any point outside it.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Why is insulin not administered orally to a diabetic class 12 biology CBSE

The total number of isomers considering both the structural class 12 chemistry CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

How do you convert from joules to electron volts class 12 physics CBSE

Define Vant Hoff factor How is it related to the degree class 12 chemistry CBSE

The first microscope was invented by A Leeuwenhoek class 12 biology CBSE




