
A capacitor and resistor are connected with an AC source as shown in figure. The capacitor is
A.
B. Zero
C.
D.
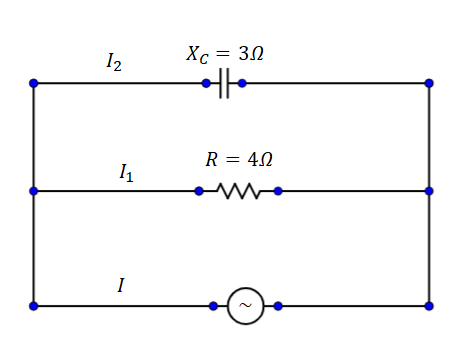

Answer
490.2k+ views
Hint:-The resultant of the capacitor resistance and the resistance of the resistor will give us the total resistance of the circuit. The current in the capacitor and in the resistor will be different as the resistance of the two elements are also different.
Formula used: The formula of the ohm's law is given by,
Complete step-by-step solution
Since the ohm's law is given by
Where V is the potential difference. I is the current in the circuit and R is the resistance in the circuit.
The current passing through the capacitor can be calculated by,
Here
Replace the value of reactance of the capacitor,
The current of the capacitor is equal to
The current flowing in the resistor of resistance of
The current flowing through the resistor of resistance
The current
The phase difference between current
Note:- The resistance offered by the capacitor in the dc circuit is infinite because the resistance offered by the capacitor is inversely proportional to the angular frequency of the circuit and the angular frequency in the dc circuit is zero.
Formula used: The formula of the ohm's law is given by,
Complete step-by-step solution
Since the ohm's law is given by
Where V is the potential difference. I is the current in the circuit and R is the resistance in the circuit.
The current passing through the capacitor can be calculated by,
Here
Replace the value of reactance of the capacitor,
The current of the capacitor is equal to
The current flowing in the resistor of resistance of
The current flowing through the resistor of resistance
The current
The phase difference between current
Note:- The resistance offered by the capacitor in the dc circuit is infinite because the resistance offered by the capacitor is inversely proportional to the angular frequency of the circuit and the angular frequency in the dc circuit is zero.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹31,500 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Class 12 Question and Answer - Your Ultimate Solutions Guide

Master Class 12 Economics: Engaging Questions & Answers for Success

Trending doubts
Give 10 examples of unisexual and bisexual flowers

Draw a labelled sketch of the human eye class 12 physics CBSE

Differentiate between homogeneous and heterogeneous class 12 chemistry CBSE

Differentiate between insitu conservation and exsitu class 12 biology CBSE

What are the major means of transport Explain each class 12 social science CBSE

Franz thinks Will they make them sing in German even class 12 english CBSE




