Question

A boy of height 1.5 m with his eye level at 1.4 m stands before a plane mirror of length 0.75 m fixed on the wall. The height of the lower edge of the mirror above the floor is 0.8 m. Then :
A. The boy will see his full image
B. The boy can't see his hair
C. The boy can't see his feet
D. The boy can neither see his hair nor his feet

Answer 1

Hint: Use the theorem for similar triangles. There are 3 triangles formed. One of the triangles has height 1.4m, the other one has height of 0.8m and then the third triangle has the height y. Using the theorem for similar triangles, equate triangles 1 and 2 and triangles 2 and 3. Thus, find y which is the length of the image which the boy can't see.

Complete answer:

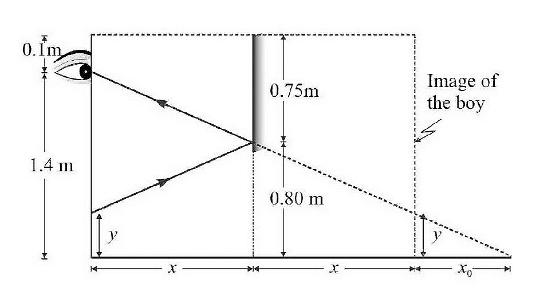
Let y be the length of the image which can't be seen by the boy.
From similar triangles theorem, we know
$\dfrac {y}{{x}_{0}}= \dfrac {0.8}{x+{x}_{0}}$
$\therefore y= \dfrac {0.8 \times { x}_{0}}{x+{x}_{0}}$ ...(1)
Now, again using the similar triangles theorem,
$\dfrac {1.4}{x+x+{x}_{0}}= \dfrac {0.8}{x+{x}_{0}}$
 $\therefore 1.4 \times (x+{x}_{0})= 0.8 \times (2x+{x}_{0})$
$\therefore { x}_{0}= \dfrac {x}{3}$
Rearranging the above equation, we get,
$x= 3 \times{ x}_{0}$ ...(2)
Now, substituting the equation. (2) in equation. (1) we get,
$y= \dfrac {0.8 \times { x}_{0}}{{3\times {x}_{0}+{x}_{0}}}$
$\therefore y =\dfrac{0.8{x}_{0}}{4{x}_{0}}$
$\therefore y= 0.2m$
Thus, the length of the image he can't see in the mirror is 0.2m i.e. he cannot see his feet.

So, the correct answer is “Option c”.

Note:
To solve such numericals, you should have the knowledge of basic mathematical tools as well. If the size of the mirror was 1.5m or greater than that then the boy could have seen the image of his full body. If the value of y was equal to the 1.5m, then the boy could not have seen his image. The minimum size of the plane mirror required to see the full image of the boy must be half the size of the boy.