
A 1.75 metre tall man, standing at the foot of a tower, sees the top of a hill 40 metres away at an elevation of
Answer
540.3k+ views
Hint: Draw the figure then use the trigonometric ratio and relate it with sides of the triangle. Then try to find the height of tower and hill using the data of the length which is given between the tower and the hill.
Complete step-by-step answer:
In the question a 1.75m tall man who is standing at the foot of the tower, sees at the top of the hill 40m away at an elevation of
Let us draw the figure first,
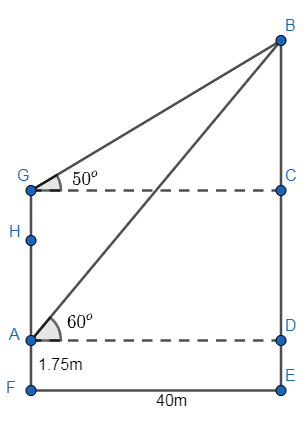
We can write or represent AF as the height of man, FG as height of the tower and BE as the height of the hill, EF and AD as the distance between tower and the hill.
So, let
BE = BD + DE
But DE = 1.75, so substituting the corresponding values, we get
Now let us consider
Now it is given
Now by using the value of
Substituting the value of
So, the value of BE is 71.03m.
Now let us consider
As we know,
By table of trigonometric table, we say that
From figure, we see that
Substituting the corresponding values, we get
So, the height of the tower is 23.43m and the height of the hill is 71.03m.
Note: Students should know how to represent trigonometric ratios as the sides of a right angled triangle. They should also be careful about the calculations to avoid any errors.
Another approach is, if the value of
Complete step-by-step answer:
In the question a 1.75m tall man who is standing at the foot of the tower, sees at the top of the hill 40m away at an elevation of
Let us draw the figure first,

We can write or represent AF as the height of man, FG as height of the tower and BE as the height of the hill, EF and AD as the distance between tower and the hill.
So, let
BE = BD + DE
But DE = 1.75, so substituting the corresponding values, we get
Now let us consider
Now it is given
Now by using the value of
Substituting the value of
So, the value of BE is 71.03m.
Now let us consider
As we know,
By table of trigonometric table, we say that
From figure, we see that
Substituting the corresponding values, we get
So, the height of the tower is 23.43m and the height of the hill is 71.03m.
Note: Students should know how to represent trigonometric ratios as the sides of a right angled triangle. They should also be careful about the calculations to avoid any errors.
Another approach is, if the value of
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 10 biology CBSE

The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Why is there a time difference of about 5 hours between class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

When and how did Canada eventually gain its independence class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE




