
How do I work with the exponential model
Answer
461.7k+ views
Hint: When we have to work with an exponential model
Complete step-by-step answer:
It is given that a function,
For exponential growth ,
We have to consider
For this we have certain features given as follows:
It is asymptotic to
It will pass through
The initial value is one.
Graph will increase without bound to the right.
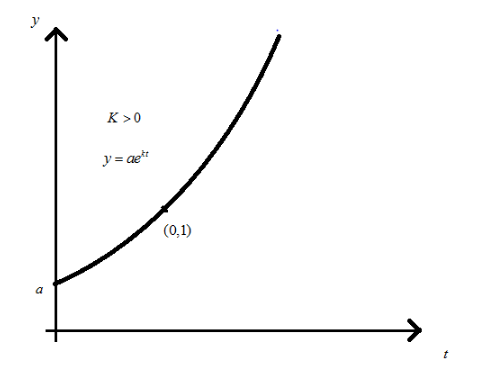
There exist some of the things where we used exponential growth include population growth model, bacterial growth model, and compound interest model.
And for exponential decay we have,
We have to consider
For this we have certain features given as follows:
It is asymptotic to
It will pass through
The initial value is one.
Graph will be decreasing , it bounded below by
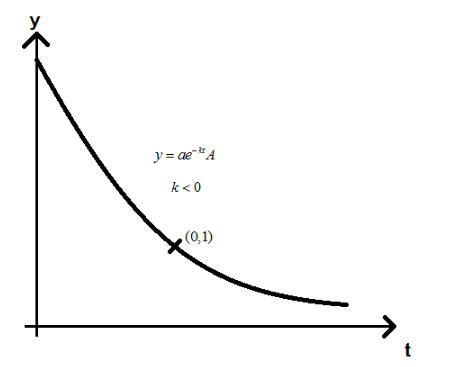
There exist some of the things where we used exponential decay including the radioactive decay model and the depreciation model.
Note: If the initial value is given to you , that is the value to get when you put
Complete step-by-step answer:
It is given that a function,
For exponential growth ,
We have to consider
For this we have certain features given as follows:
It is asymptotic to
It will pass through
The initial value is one.
Graph will increase without bound to the right.

There exist some of the things where we used exponential growth include population growth model, bacterial growth model, and compound interest model.
And for exponential decay we have,
We have to consider
For this we have certain features given as follows:
It is asymptotic to
It will pass through
The initial value is one.
Graph will be decreasing , it bounded below by

There exist some of the things where we used exponential decay including the radioactive decay model and the depreciation model.
Note: If the initial value is given to you , that is the value to get when you put
Latest Vedantu courses for you
Grade 10 | CBSE | SCHOOL | English
Vedantu 10 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Write examples of herbivores carnivores and omnivo class 10 biology CBSE

Difference between mass and weight class 10 physics CBSE

List out three methods of soil conservation

Leap year has days A 365 B 366 C 367 D 368 class 10 maths CBSE

How does Tommy describe the old kind of school class 10 english CBSE




