
Water is filled to a height
Answer
505.5k+ views
3 likes
Hint: Pressure is defined as force per unit area. In other words, force is defined as the product of pressure and the area, on which the pressure is applied. A small strip of the length of the dam wall is considered at a particular distance from the surface of the vertical dam. Pressure on this small strip of the length of the dam wall and thus, the force on this small strip is determined. Force on the dam is nothing but the integral of this small force, acting on the small strip of the length of the dam wall.
Formula used:
Complete step-by-step solution
We are given that water is filled to a height
Firstly, let us consider the figure given below. Clearly,
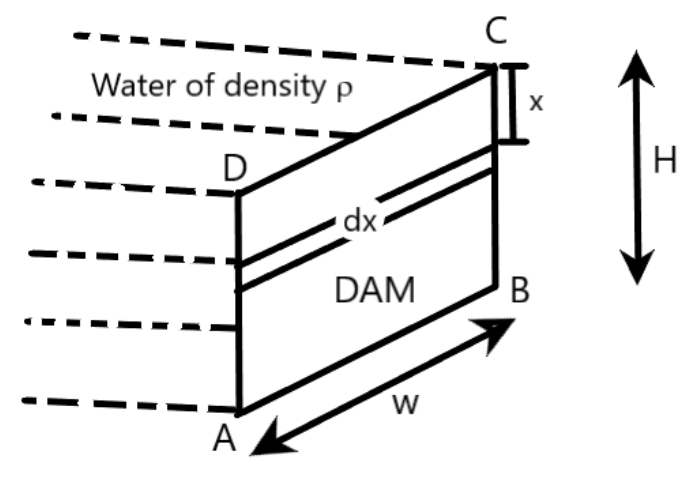
Now, let us consider a small strip of the length of the dam wall, at a distance
where
Let this be equation 1.
Also, if
where
Let this be equation 2.
Now, we know that the horizontal force on the small strip of the dam wall is equal to the product of pressure applied on this strip by the water behind the wall and the area of cross-section of the small strip of the dam wall. Clearly, if
where
Let this be equation 3.
Substituting equation 1 and equation 2 in equation 3, we have
Let this be equation 4.
Now, taking the integral of equation 4 from zero to
where
Let this be equation 5.
Clearly, from equation 5, the resultant force acting on the dam wall is equal to
Therefore, the correct answer is option
Note: Students need to adapt this method of taking small cross-sectional areas whenever they are dealing with problems involving volume, area, pressure, or force related to fluids. Determining the required parameter on these small cross-sectional areas, and then integrating them over the necessary values will provide appropriate results, as done in the above solution. Thus students need to be thorough with integral formulas too. The integral formula used in this solution is given as follows:
Formula used:
Complete step-by-step solution
We are given that water is filled to a height
Firstly, let us consider the figure given below. Clearly,

Now, let us consider a small strip of the length of the dam wall, at a distance
where
Let this be equation 1.
Also, if
where
Let this be equation 2.
Now, we know that the horizontal force on the small strip of the dam wall is equal to the product of pressure applied on this strip by the water behind the wall and the area of cross-section of the small strip of the dam wall. Clearly, if
where
Let this be equation 3.
Substituting equation 1 and equation 2 in equation 3, we have
Let this be equation 4.
Now, taking the integral of equation 4 from zero to
where
Let this be equation 5.
Clearly, from equation 5, the resultant force acting on the dam wall is equal to
Therefore, the correct answer is option
Note: Students need to adapt this method of taking small cross-sectional areas whenever they are dealing with problems involving volume, area, pressure, or force related to fluids. Determining the required parameter on these small cross-sectional areas, and then integrating them over the necessary values will provide appropriate results, as done in the above solution. Thus students need to be thorough with integral formulas too. The integral formula used in this solution is given as follows:
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




