
Two charged particles
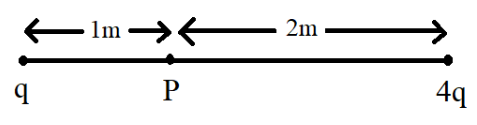
The force on charge
A.
B.
C.
D. Zero
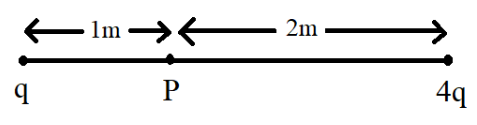
Answer
428.4k+ views
1 likes
Hint: When a charged particle comes in the range of other point charges in that region then it experiences a force on it due to the point charges present in that region. The formula for the electrostatic force due to other charged particles is applied along with the concept of Coulomb’s law in order to determine the total force on charge
Formula used:
The Coulomb’s force is given by the equation:
Where,
Complete step by step answer:
The above problem revolves around the concept of Coulomb’s law. In order to find out the force on the charged particle
The electrostatic force applied on the test charge by a point charge separated by a distance is given by a formula given by Coulomb’s law and hence this equation is:
Let us now extract the data given in the question. The distances between the respective point charges and the charge
Given,
When a charged particle is brought in the range of other point charges having some charge then this charged particle is said to get affected. The particle considered here, that is, charged particle
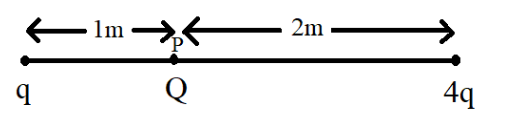
force due to the point charges
Since the force is a vector quantity it is said to have magnitude and some direction. Hence the direction is also considered here. Since the force is said to be repulsive in nature then we can say that the force due to point charge
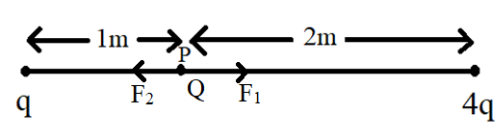
The
From the equation (
Hence,
We now apply the equation (
Now, we apply the equation (
We now substitute equations (
By taking out the
By substituting the given values we get:
By solving further we get:
Hence, the total force exerted on charge
Therefore, the correct option is option D.
Note: A common error which is observed in the above problem is that the direction of the electrostatic force exerted on
Formula used:
The Coulomb’s force is given by the equation:
Where,
Complete step by step answer:
The above problem revolves around the concept of Coulomb’s law. In order to find out the force on the charged particle
The electrostatic force applied on the test charge by a point charge separated by a distance is given by a formula given by Coulomb’s law and hence this equation is:
Let us now extract the data given in the question. The distances between the respective point charges and the charge
Given,
When a charged particle is brought in the range of other point charges having some charge then this charged particle is said to get affected. The particle considered here, that is, charged particle
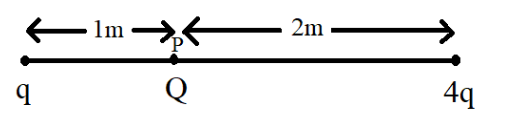
force due to the point charges
Since the force is a vector quantity it is said to have magnitude and some direction. Hence the direction is also considered here. Since the force is said to be repulsive in nature then we can say that the force due to point charge

The
From the equation (
Hence,
We now apply the equation (
Now, we apply the equation (
We now substitute equations (
By taking out the
By substituting the given values we get:
By solving further we get:
Hence, the total force exerted on charge
Therefore, the correct option is option D.
Note: A common error which is observed in the above problem is that the direction of the electrostatic force exerted on
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

How do you convert from joules to electron volts class 12 physics CBSE

Why should a magnesium ribbon be cleaned before burning class 12 chemistry CBSE

Why did French artist Frederic sorrieu prepare a series class 12 social science CBSE

Give 10 examples of unisexual and bisexual flowers




