
How is trigonometric substitution different from u substitution?
Answer
481.8k+ views
Hint: In mathematics, trigonometric substitution is the substitution of trigonometric functions for other expressions. It is a technique for evaluating integrals with the help of calculus concepts. A trigonometric substitution is used for integrals of the form
Complete step-by-step answer:
Let us first consider the trigonometric substitution.
Here, we will use the two most important concepts of trigonometry that are the Pythagoras theorem and its identities.
Pythagoras theorem relates two sides of the right-angle triangle to the hypotenuse.
Let us understand with the help of a figure.
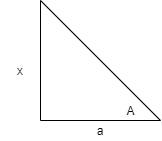
In the above figure, two sides are x and a, and hypotenuse will be as follow:
According to Pythagoras theorem,
From the above figure, we can also write the trigonometric ratios of angle A.
For example,
We can also write as,
Put the value of x in
So,
That is equal to,
Then find the integral.
Now, let us consider u-substitution.
We use this technique when we have something like
Here, we can see two functions:
And we know the derivative concepts,
So, we will take the value of u as
Then
So that,
Then find the integral.
Using u-substitution, the calculation is easier than trigonometric substitution.
Note:
While these two techniques may differ, they both serve the same purpose. And the purpose is to reduce an integral to a simpler form. So, we can use the basic techniques of integral. A trigonometric substitution is used for integrals of the form
Complete step-by-step answer:
Let us first consider the trigonometric substitution.
Here, we will use the two most important concepts of trigonometry that are the Pythagoras theorem and its identities.
Pythagoras theorem relates two sides of the right-angle triangle to the hypotenuse.
Let us understand with the help of a figure.

In the above figure, two sides are x and a, and hypotenuse will be as follow:
According to Pythagoras theorem,
From the above figure, we can also write the trigonometric ratios of angle A.
For example,
We can also write as,
Put the value of x in
So,
That is equal to,
Then find the integral.
Now, let us consider u-substitution.
We use this technique when we have something like
Here, we can see two functions:
And we know the derivative concepts,
So, we will take the value of u as
Then
So that,
Then find the integral.
Using u-substitution, the calculation is easier than trigonometric substitution.
Note:
While these two techniques may differ, they both serve the same purpose. And the purpose is to reduce an integral to a simpler form. So, we can use the basic techniques of integral. A trigonometric substitution is used for integrals of the form
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




