
How many triangles can be formed by joining the vertices of a hexagon?
A. 31
B. 25
C. 20
D. 60
Answer
512.4k+ views
Hint: As we know that hexagon has
Complete step by step solution:
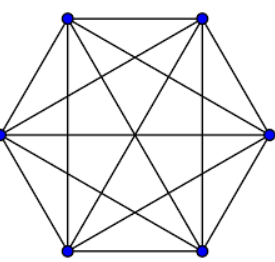
The number of vertices in a hexagon is
The number of vertices in a triangle is
That means to make a triangle we need
So,
By applying the formula of combination:
Therefore,
By using the values of factorial terms:
We have,
Replace
Note:
These types of questions always use a combination concept for solving the problem.
Some important definitions you should know
Vertices mean the point where
Combination: Any of the ways we can combine things when the order does not matter.
Combination formula: The formula of combination is
The factorial function
The representation of factorial is
Alternatively, you can draw the figure and count the triangles but it becomes complicated.
Complete step by step solution:

The number of vertices in a hexagon is
The number of vertices in a triangle is
That means to make a triangle we need
So,
By applying the formula of combination:
Therefore,
By using the values of factorial terms:
We have,
Replace
Note:
These types of questions always use a combination concept for solving the problem.
Some important definitions you should know
Vertices mean the point where
Combination: Any of the ways we can combine things when the order does not matter.
Combination formula: The formula of combination is
The factorial function
The representation of factorial is
Alternatively, you can draw the figure and count the triangles but it becomes complicated.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




