
Three long straight wires in the XZ-plane, each carrying

(A)
(B)
(C)
(D)

Answer
497.1k+ views
Hint To solve this question, we have to use the formula for the magnetic field due to an infinitely long carrying wire. And for getting the direction, we need to use the right hand thumb rule.
The formula which is used in solving this question is given by
Complete step by step answer
Let us consider a point P on the x-axis at a distance of

We know that the magnetic field due to an infinitely long wire is given by
So we need to calculate the perpendicular distance of the point P from each of the wires A, B and C.
For wire A:
Consider the following figure.
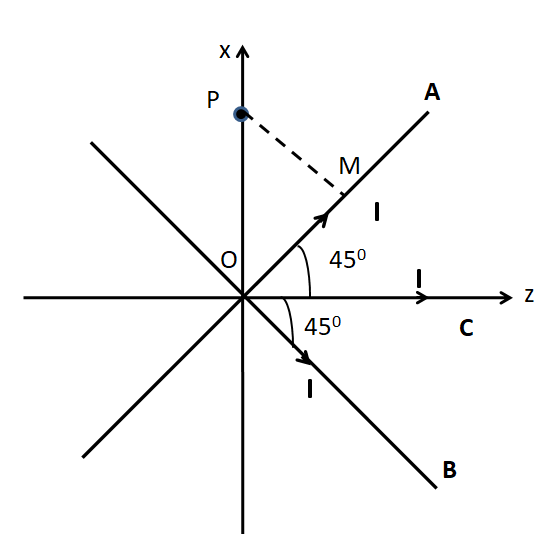
From the figure, we see that the perpendicular distance of the point P is
In the triangle
So we get
So from (1) the magnetic field due to wire A at the point P is
From (2)
By the right hand thumb rule, the direction of this field is along the positive y-axis. So in terms of the unit vector we have
Similarly, the magnetic field due to the wire B at the point P
Also, the magnetic field at P due to wire C is
So the net magnetic field at point P is
From (3) (4) and (5)
On simplifying we get
Thus, this is the required magnetic field as a function of
Hence, the correct answer is option (C).
Note
The given set of axes is not the standard set of coordinate axes. So, for getting the direction of the positive y-axis, we use the right hand thumb rule. We should not assume the direction by ourselves.
The formula which is used in solving this question is given by
Complete step by step answer
Let us consider a point P on the x-axis at a distance of

We know that the magnetic field due to an infinitely long wire is given by
So we need to calculate the perpendicular distance of the point P from each of the wires A, B and C.
For wire A:
Consider the following figure.

From the figure, we see that the perpendicular distance of the point P is
In the triangle
So we get
So from (1) the magnetic field due to wire A at the point P is
From (2)
By the right hand thumb rule, the direction of this field is along the positive y-axis. So in terms of the unit vector we have
Similarly, the magnetic field due to the wire B at the point P
Also, the magnetic field at P due to wire C is
So the net magnetic field at point P is
From (3) (4) and (5)
On simplifying we get
Thus, this is the required magnetic field as a function of
Hence, the correct answer is option (C).
Note
The given set of axes is not the standard set of coordinate axes. So, for getting the direction of the positive y-axis, we use the right hand thumb rule. We should not assume the direction by ourselves.
Recently Updated Pages
Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Biology: Engaging Questions & Answers for Success

Master Class 12 Physics: Engaging Questions & Answers for Success

Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Trending doubts
Which one of the following is a true fish A Jellyfish class 12 biology CBSE

What is the Full Form of PVC, PET, HDPE, LDPE, PP and PS ?

What are saprophytes Give two examples class 12 biology CBSE

How do you convert from joules to electron volts class 12 physics CBSE

Mirage is a phenomena due to A Refraction of light class 12 physics CBSE

Why did French artist Frederic sorrieu prepare a series class 12 social science CBSE




