
The velocity-displacement graph of a particle is shown in figure.
a) Write the relation between v and x.
b) Obtain the relation between acceleration and displacement and plot it.
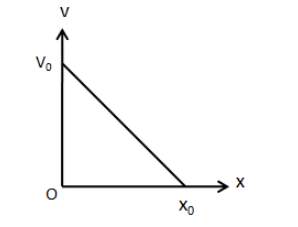

Answer
492.3k+ views
2 likes
Hint:Express the angle made by the straight line with the horizontal axis. Then assume the position of the particle at any point of the straight line and then express the angle made by this straight line with the horizontal axis. Comparing the two equations, we will get the relation between v and x. The derivative of velocity with respect to time is the acceleration of the particle.
Complete step by step answer:
(a) We have given the initial velocity
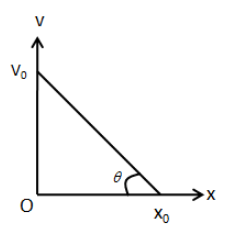
From the above figure, we can express
Now, we assume at any instant of time, the particle has velocity v and displacement x. The position of the particle is shown by the point A in the following figure.
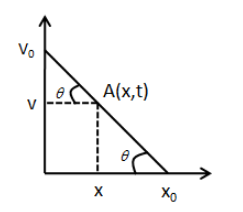
We can express
From equation (1) and (2), we can write,
This is the relation between v and x.
(b) We know that the acceleration is the derivative of velocity of the particle. Therefore, we can write.
We have the expression for velocity obtained in part (a) of the solution. Therefore, the acceleration of the particle is,
Using equation (3), we can rewrite the above equation as,
From the above expression, if the displacement of the particle x is zero. Then the acceleration of the particle is
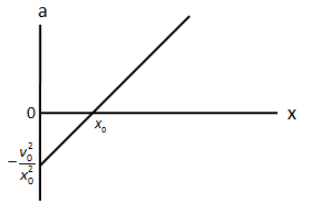
The acceleration is zero when the displacement
Note: In equation (1) and (2), the angle
Complete step by step answer:
(a) We have given the initial velocity

From the above figure, we can express
Now, we assume at any instant of time, the particle has velocity v and displacement x. The position of the particle is shown by the point A in the following figure.

We can express
From equation (1) and (2), we can write,
This is the relation between v and x.
(b) We know that the acceleration is the derivative of velocity of the particle. Therefore, we can write.
We have the expression for velocity obtained in part (a) of the solution. Therefore, the acceleration of the particle is,
Using equation (3), we can rewrite the above equation as,
From the above expression, if the displacement of the particle x is zero. Then the acceleration of the particle is

The acceleration is zero when the displacement
Note: In equation (1) and (2), the angle
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE

Give two reasons to justify a Water at room temperature class 11 chemistry CBSE




