
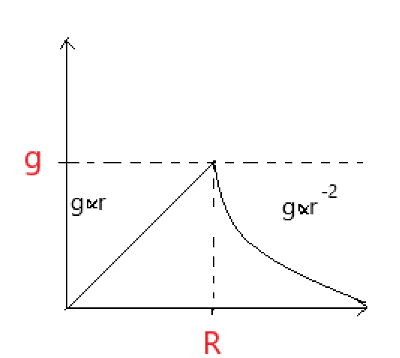
The variation of g with height or depth (r) is shown correctly by the graph in figure (where R is the radius of the earth).
A.
B.
C.
D.




Answer
526.8k+ views
Hint: For this question we start with the equation for g with respect to distance r from the center of the earth with mass M and radius R that is
Complete Step-by-Step solution:
We know that the acceleration due to gravity is denoted by the symbol g and is defined as the acceleration of an object because of the force acting on that object by the gravitational field of Earth. This force is written as
Here m is the mass of the object. If
This also says that g is the force on the unit mass. The g is independent of the mass of the body and can be accurately calculated in various ways.
Now we will try to see the variation of g with respect to height h. So let us assume an object of mass m, then the measure of the gravitational field strength
Now take the object at the surface of the earth that is r=R as shown in figure 1.
Here R is the radius of the earth. We get
Now we assume that the object is at a height H from the surface of the earth as shown in figure 2.

Now dividing equation (2) by (1) we get
This expression is used when
But when
From these we can conclude that
Region 1: When
Region 2: When
So the graph we get is shown in figure 3
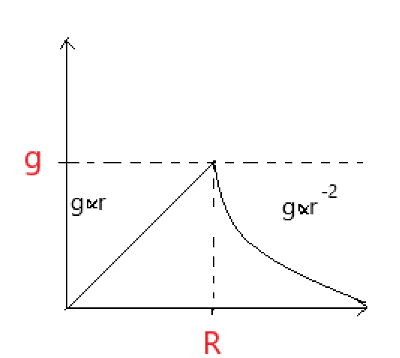
Figure 3
Hence option A is correct.
Note: We can also solve this question in a different way that is we get the expression of g with respect to r when
Complete Step-by-Step solution:
We know that the acceleration due to gravity is denoted by the symbol g and is defined as the acceleration of an object because of the force acting on that object by the gravitational field of Earth. This force is written as
Here m is the mass of the object. If
This also says that g is the force on the unit mass. The g is independent of the mass of the body and can be accurately calculated in various ways.
Now we will try to see the variation of g with respect to height h. So let us assume an object of mass m, then the measure of the gravitational field strength
Now take the object at the surface of the earth that is r=R as shown in figure 1.

Figure 1
Here R is the radius of the earth. We get
Now we assume that the object is at a height H from the surface of the earth as shown in figure 2.

Figure 2
Now dividing equation (2) by (1) we get
This expression is used when
But when
From these we can conclude that
Region 1: When
Region 2: When
So the graph we get is shown in figure 3

Figure 3
Hence option A is correct.
Note: We can also solve this question in a different way that is we get the expression of g with respect to r when
Recently Updated Pages
Master Class 12 Business Studies: Engaging Questions & Answers for Success

Master Class 12 English: Engaging Questions & Answers for Success

Master Class 12 Economics: Engaging Questions & Answers for Success

Master Class 12 Social Science: Engaging Questions & Answers for Success

Master Class 12 Maths: Engaging Questions & Answers for Success

Master Class 12 Chemistry: Engaging Questions & Answers for Success

Trending doubts
The Equation xxx + 2 is Satisfied when x is Equal to Class 10 Maths

Gautam Buddha was born in the year A581 BC B563 BC class 10 social science CBSE

Fill the blanks with proper collective nouns 1 A of class 10 english CBSE

Why is there a time difference of about 5 hours between class 10 social science CBSE

What is the median of the first 10 natural numbers class 10 maths CBSE

Change the following sentences into negative and interrogative class 10 english CBSE




