Question

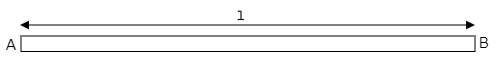
The uniform rod of length $l$ and mass $m$ is free to rotate in the vertical plane about $A$. The rod initially in a horizontal position is released. The initial angular acceleration of the rod is (Moment of inertia of rod about $A$ is $\dfrac{{m{l^2}}}{3}$).

(A) $\dfrac{{3g}}{{2l}}$
(B) $\dfrac{{2l}}{{3g}}$
(C) $\dfrac{{3g}}{{2{l^2}}}$
(D) $\dfrac{l}{2}$

Answer 1

Hint
The initial angular acceleration of the rod is determined by the formula which shows the relation between the torque, moment of inertia and angular acceleration of the rod. But here the torque is written as the product of the mass of the rod, acceleration due to gravity and length of the rod.
The torque of the rod is given by,
$\Rightarrow \tau = I\alpha $
Where, $\tau $ is the torque of the rod, $I$ is the moment of inertia of the rod and $\alpha $ is the angular velocity of the rod.

Complete step by step answer
Given that, The moment of inertia of the rod about $A$ is, $I = \dfrac{{m{l^2}}}{3}$.
Now, The torque of the rod is given by,
$\Rightarrow \tau = I\alpha \,...................\left( 1 \right)$
So, the torque is given by,
$\Rightarrow \tau = m \times g \times \dfrac{l}{2}$
Where, $m$ is the mass of the rod, $g$ is the acceleration due to gravity and $l$ is the length of the rod.
By substituting the torque value in the equation (1), then the equation (1) is written as,
$\Rightarrow \dfrac{{m \times g \times l}}{2} = I\alpha \,...................\left( 2 \right)$
By substituting the moment of inertia in the equation (2), then
$\Rightarrow \dfrac{{m \times g \times l}}{2} = \dfrac{{m{l^2}}}{3}\alpha $
By keeping the angular acceleration in one side and the other terms in other side, then
$\Rightarrow \dfrac{{m \times g \times l \times 3}}{{2 \times m \times {l^2}}} = \alpha $
By cancelling the same terms, then the above equation is written as,
$\Rightarrow \alpha = \dfrac{{3g}}{{2l}}$.
Hence, the option (A) is the correct answer.

Note
Generally, the torque is defined as the product of the force and the perpendicular distance. But here the rod is allowed to free fall, so the force acted on it is given by the product of the mass of the rod and the acceleration due to gravity. Then the total torque is given by the product of the force due to gravitation and the half the length of the rod, because the force due to gravitation acts at the centre of the rod.