
The three vertices of a parallelogram are (3, 4), (3, 8) and (9, 8). Find the fourth vertex.
Answer
528.3k+ views
5 likes
Hint: Assume the coordinate of the fourth vertex of parallelogram ABCD to be (x,y). We know that the diagonal of a parallelogram bisects each other. Since ABCD is a parallelogram, the diagonals must bisect each other. We know that the midpoint(x,y) of A
Complete step-by-step answer:
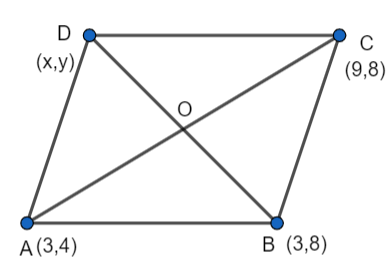
Let the coordinate of the fourth vertex D be (x,y).
We know that the diagonals of a parallelogram bisect each other. Since ABCD is a parallelogram, the diagonals must bisect each other.
For diagonal AC, O is its midpoint.
We know the formula that the midpoint(x,y) of A
As O is the midpoint of the diagonal AC, we can find its coordinates using the midpoint formula.
We have, A = (3,4) and C = (9,8),
O =
As O is the midpoint of the diagonal BD, we can find its coordinates using the midpoint formula.
We have, B = (3,8) and D = (x,y),
O =
Comparing equation (1) and equation (2), we get
Solving equation (3), we get
Solving equation (4), we get
The values of x and y are 4 and 9 respectively.
So, D = (9,4).
Hence, the fourth vertex is (9,4).
Note: We can also solve this question using the distance formula.

Let the fourth vertex of the parallelogram be (x,y).
We know that the opposite sides of a parallelogram are equal to each other.
So, AB = CD and BC = AD.
AB =
BC =
CD =
AD =
From equation (1) and equation (3), we get
From equation (2) and equation (4), we get
We have two equations and two variables. On solving equation (5) and equation (6), we get
x=9 and y=4.
Hence, the fourth vertex is (9,4).
Complete step-by-step answer:

Let the coordinate of the fourth vertex D be (x,y).
We know that the diagonals of a parallelogram bisect each other. Since ABCD is a parallelogram, the diagonals must bisect each other.
For diagonal AC, O is its midpoint.
We know the formula that the midpoint(x,y) of A
As O is the midpoint of the diagonal AC, we can find its coordinates using the midpoint formula.
We have, A = (3,4) and C = (9,8),
O =
As O is the midpoint of the diagonal BD, we can find its coordinates using the midpoint formula.
We have, B = (3,8) and D = (x,y),
O =
Comparing equation (1) and equation (2), we get
Solving equation (3), we get
Solving equation (4), we get
The values of x and y are 4 and 9 respectively.
So, D = (9,4).
Hence, the fourth vertex is (9,4).
Note: We can also solve this question using the distance formula.

Let the fourth vertex of the parallelogram be (x,y).
We know that the opposite sides of a parallelogram are equal to each other.
So, AB = CD and BC = AD.
AB =
BC =
CD =
AD =
From equation (1) and equation (3), we get
From equation (2) and equation (4), we get
We have two equations and two variables. On solving equation (5) and equation (6), we get
x=9 and y=4.
Hence, the fourth vertex is (9,4).
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE




