
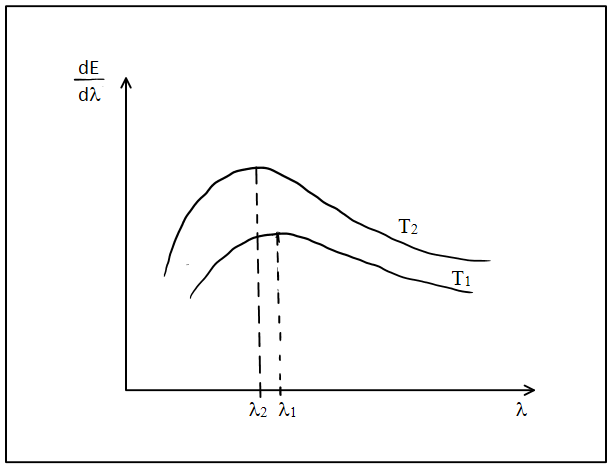
The spectral emissive power
(A) 3
(B)
(C)
(D)

Answer
509.4k+ views
3 likes
Hint: The area under enclosed by the graph formed between the quantities
Complete step by step solution:
The total area under the graph formed between the quantities
Given that total emissive power at temperature T1 is A,
Using Stefan's law we can say that :
Given that total emissive power at temperature T2 is 9A,
Using Stefan's law we can say that
Dividing these two equations, we get :
Cancelling A and σ on both sides, we get :
Cross multiplication gives,
Raising power
Now, using wien's displacement law :
Substituting the value of T2, we get :
Cancelling T1 on both sides :
Therefore the correct answer is option : (D)
Note: Before solving the problem, the student needs to be able to understand the different concepts of Radiation like Stefan’s law, Wien's Displacement law and the terms like total emissive power and spectral emissive power. A common mistake that students make while solving questions involving concepts of radiation is not being able to differentiate between spectral emissive power and total emissive power. While total emissive power is the intensity of radiation emitted by a body, spectral emissive power is the intensity of radiation emitted by a body per unit wavelength.
Complete step by step solution:
The total area under the graph formed between the quantities
Given that total emissive power at temperature T1 is A,
Using Stefan's law we can say that :
Given that total emissive power at temperature T2 is 9A,
Using Stefan's law we can say that
Dividing these two equations, we get :
Cancelling A and σ on both sides, we get :
Cross multiplication gives,
Raising power
Now, using wien's displacement law :
Substituting the value of T2, we get :
Cancelling T1 on both sides :
Therefore the correct answer is option : (D)
Note: Before solving the problem, the student needs to be able to understand the different concepts of Radiation like Stefan’s law, Wien's Displacement law and the terms like total emissive power and spectral emissive power. A common mistake that students make while solving questions involving concepts of radiation is not being able to differentiate between spectral emissive power and total emissive power. While total emissive power is the intensity of radiation emitted by a body, spectral emissive power is the intensity of radiation emitted by a body per unit wavelength.
Recently Updated Pages
Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Class 11 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
The non protein part of an enzyme is a A Prosthetic class 11 biology CBSE

Which of the following blood vessels in the circulatory class 11 biology CBSE

What is a zygomorphic flower Give example class 11 biology CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

The deoxygenated blood from the hind limbs of the frog class 11 biology CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE




