
The number of vibrational degrees of freedom for a
a) 4
b) 5
c) 6
d) 9
Answer
513.6k+ views
1 likes
Hint: Though carbon dioxide is a triatomic molecule, it is not arranged in a triangular fashion. It is a linear molecule with all the three atoms arranged in the same line. Hence we would use the relation between the number of linkages of the atoms of the molecule to determine the vibrational degrees of freedom for a
Complete step-by-step answer:
Let us first understand what does degrees of freedom in a molecule means,
Degrees of freedom of a system or a molecule is the number of independent ways in which the particles of the system can absorb energy.
It can also be defined as the number of coordinates required to specify the position of the constituent particles of the system minus the number of independent relation, i.e.
The above relation that we obtained is for total degrees of freedom of a molecule at moderate temperatures. But at higher temperatures the molecule possesses an additional degree of freedom that is a vibrational degree of freedom. Hence, now the number of degrees of freedom of a molecule with N particles is given by,
A
Hence the carbon dioxide molecule has 4 vibrational degrees of freedom. Hence the correct answer is option a.
Note:
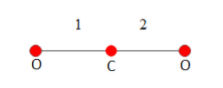
In a symmetric carbon dioxide molecule as shown above, the two oxygen molecules vibrate about the carbon molecule. Each vibrational motion has both kinetic and potential energies. So one degree of freedom of vibrational motion is taken as two. Since the above molecule vibrates along two linkages we can directly conclude it has four degrees of freedom.
Complete step-by-step answer:
Let us first understand what does degrees of freedom in a molecule means,
Degrees of freedom of a system or a molecule is the number of independent ways in which the particles of the system can absorb energy.
It can also be defined as the number of coordinates required to specify the position of the constituent particles of the system minus the number of independent relation, i.e.
The above relation that we obtained is for total degrees of freedom of a molecule at moderate temperatures. But at higher temperatures the molecule possesses an additional degree of freedom that is a vibrational degree of freedom. Hence, now the number of degrees of freedom of a molecule with N particles is given by,
A
Hence the carbon dioxide molecule has 4 vibrational degrees of freedom. Hence the correct answer is option a.
Note:

In a symmetric carbon dioxide molecule as shown above, the two oxygen molecules vibrate about the carbon molecule. Each vibrational motion has both kinetic and potential energies. So one degree of freedom of vibrational motion is taken as two. Since the above molecule vibrates along two linkages we can directly conclude it has four degrees of freedom.
Latest Vedantu courses for you
Grade 10 | MAHARASHTRABOARD | SCHOOL | English
Vedantu 10 Maharashtra Pro Lite (2025-26)
School Full course for MAHARASHTRABOARD students
₹33,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




