
The number of corner of O-atoms shared per tetrahedron in 2D- silicate is:
[A] 3
[B] 4
[C] 2
[D] 5
Answer
490.2k+ views
1 likes
Hint: Silicates contain a silicon atom that is covalently bonded to four oxygen atoms. 2D silicates are also known as sheet silicates. They form a sheet like structure where the structure is formed by sharing of oxygen atoms.
Complete step by step answer:
Before discussing the answer, we will discuss what silicates are and their varieties.
We know that all silicates contain tetrahedral
There are ortho silicates in which none of the oxygen atoms are shared, pyro silicates in which one oxygen atom is shared and there are many more such possibilities.
Now let us discuss 2D silicates.
These silicates are also known as sheet silicate or phyllosilicate. In this type of silicates, the
The simplest sheet silicate is
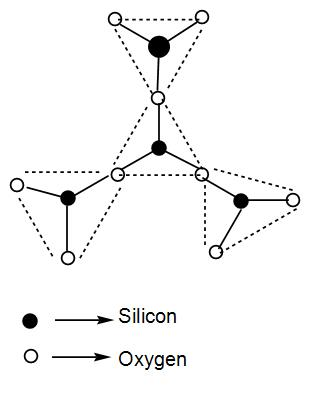
The general formula of phyllo or 2d silicates is
Here, all the three oxygen atoms of the tetrahedron are shared with other similar units. In the above diagram we have not drawn the sheet structure but just one unit cell of the complete structure where all the three oxygen atoms will be shared.
We can understand from the above discussion that the number of corners of O-atoms shared per tetrahedron in 2D- silicate is 3.
So, the correct answer is “Option A”.
Note: Example of a sheet silicate is Soapstone. Its formula is
Special case of sheet silicates is Mica. Here, one silicon atom is replaced by an aluminium atom. Instead of
Complete step by step answer:
Before discussing the answer, we will discuss what silicates are and their varieties.
We know that all silicates contain tetrahedral
There are ortho silicates in which none of the oxygen atoms are shared, pyro silicates in which one oxygen atom is shared and there are many more such possibilities.
Now let us discuss 2D silicates.
These silicates are also known as sheet silicate or phyllosilicate. In this type of silicates, the
The simplest sheet silicate is

The general formula of phyllo or 2d silicates is
Here, all the three oxygen atoms of the tetrahedron are shared with other similar units. In the above diagram we have not drawn the sheet structure but just one unit cell of the complete structure where all the three oxygen atoms will be shared.
We can understand from the above discussion that the number of corners of O-atoms shared per tetrahedron in 2D- silicate is 3.
So, the correct answer is “Option A”.
Note: Example of a sheet silicate is Soapstone. Its formula is
Special case of sheet silicates is Mica. Here, one silicon atom is replaced by an aluminium atom. Instead of
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
State and prove Bernoullis theorem class 11 physics CBSE

What are Quantum numbers Explain the quantum number class 11 chemistry CBSE

Write the differences between monocot plants and dicot class 11 biology CBSE

Who built the Grand Trunk Road AChandragupta Maurya class 11 social science CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

State the laws of reflection of light




