
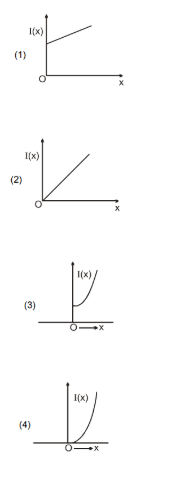
The moment of inertia of a solid sphere, about an axis parallel to its diameter and at a distance of x from it, is I(x)'. Which one of the graphs represents the variation of I(x) with x correctly?

Answer
518.4k+ views
2 likes
Hint: Moment of inertia is the rotational analog of mass. Moment of inertia is measured by taking the second moment of mass with respect to distance from an axis. We have to find the equation of the graph I(x) v/s x and by comparing it with the general form of equations for conic sections we can find its shape (I.e. whether it is linear, parabolic etc.). Once the equation is known we can find the shape of the graph.
Formula used:
Complete step by step answer:
Moment of inertia of the solid sphere about an axis parallel to its diameter and at a distance of x from it can be found out by using parallel axis theorem.
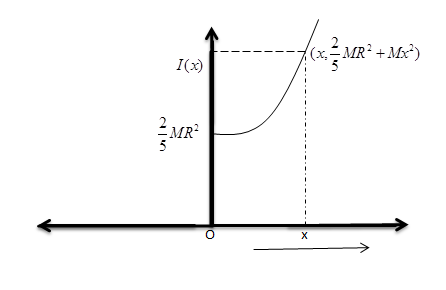
This is in the form of
Therefore (3) is the correct option.
Additional Information:
Parallel Axis Theorem: The moment of inertia of a body about any axis is equal to the sum of its moment of inertia about a parallel axis through its center of gravity and the product of the mass of the body and the square of the distance between the two axes.
Perpendicular Axis theorem: The moment of inertia of a plane laminar body about an axis perpendicular to the plane is equal to the sum of the moments of inertia about two mutually perpendicular axes in the plane of the lamina such that the three mutually perpendicular axes have a common point of intersection.
Note: When the value of x is zero, the moment of inertia of the solid sphere
Using
Formula used:
Complete step by step answer:
Moment of inertia of the solid sphere about an axis parallel to its diameter and at a distance of x from it can be found out by using parallel axis theorem.

This is in the form of
Therefore (3) is the correct option.
Additional Information:
Parallel Axis Theorem: The moment of inertia of a body about any axis is equal to the sum of its moment of inertia about a parallel axis through its center of gravity and the product of the mass of the body and the square of the distance between the two axes.
Perpendicular Axis theorem: The moment of inertia of a plane laminar body about an axis perpendicular to the plane is equal to the sum of the moments of inertia about two mutually perpendicular axes in the plane of the lamina such that the three mutually perpendicular axes have a common point of intersection.
Note: When the value of x is zero, the moment of inertia of the solid sphere
Using
Latest Vedantu courses for you
Grade 9 | CBSE | SCHOOL | English
Vedantu 9 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹37,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Net gain of ATP in glycolysis a 6 b 2 c 4 d 8 class 11 biology CBSE




