
The moment of inertia of a hollow sphere (mass M) of inner radius R and outer radius 2R, having material of uniform density, about a diametric axis is:
A.
B.
C.
D. None
Answer
509.1k+ views
1 likes
Hint: Recall that a hollow sphere does not enclose any mass within its boundary. Therefore, the only source of mass which contributes to the moment of inertia of such a sphere is the mass enclosed between the inner and outer radius of the sphere. In such a case, assuming that the density of the sphere remains uniform throughout this enclosed region, first determine an expression for the density of this region taking it as mass per volume of the region, and then determine the mass of the enclosed region by taking the difference in the mass encompassed by the two radii.
From this, the moment of inertia can be determined by taking the difference of the moment of inertia about the diametric axis between the inner and outer radii and corresponding masses to ultimately arrive at the required solution.
Formula used:
Moment of inertia of hollow spherical shell
Complete step-by-step answer:
The moment of inertia of the hollow sphere comes from the region enclosed between the inner and outer radius of the sphere, since all mass of the sphere lies in this enclosed region.
We now need to find
Let us assume that the density of the sphere over this region between R and 2R is uniform, and can be given as:
Now,
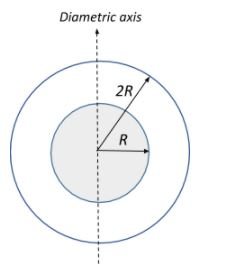
Similarly,
Therefore, from the equation for the moment of inertia:
Therefore, the correct choice would be: A.
So, the correct answer is “Option A”.
Note: Always remember that the moment of inertia does not only depend on the shape of the object but also on the position and orientation of the axis of rotation and the distribution of mass of the body about the axis of rotation. If our spherical shell was variably dense across the diametric axis, then we would have to find the mass of the sphere across every differently dense region and cumulatively add them up together to get the mass of the enclosed region.
From this, the moment of inertia can be determined by taking the difference of the moment of inertia about the diametric axis between the inner and outer radii and corresponding masses to ultimately arrive at the required solution.
Formula used:
Moment of inertia of hollow spherical shell
Complete step-by-step answer:
The moment of inertia of the hollow sphere comes from the region enclosed between the inner and outer radius of the sphere, since all mass of the sphere lies in this enclosed region.
We now need to find
Let us assume that the density of the sphere over this region between R and 2R is uniform, and can be given as:
Now,

Similarly,
Therefore, from the equation for the moment of inertia:
Therefore, the correct choice would be: A.
So, the correct answer is “Option A”.
Note: Always remember that the moment of inertia does not only depend on the shape of the object but also on the position and orientation of the axis of rotation and the distribution of mass of the body about the axis of rotation. If our spherical shell was variably dense across the diametric axis, then we would have to find the mass of the sphere across every differently dense region and cumulatively add them up together to get the mass of the enclosed region.
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE




