
The moment of inertia of a disc of radius
Answer
504.9k+ views
Hint
Torque is the force that is responsible for making an object rotate about an axis. Depending on the position of the axis and the distance of the force acting, the value of torque may vary.
Formula used:
Complete step by step answer
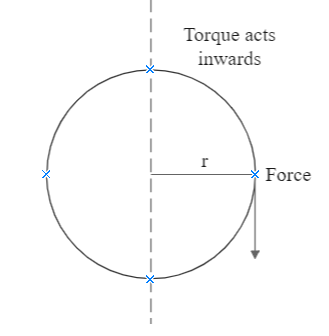
In this question, we are provided with a circular disc. A string is attached on the circumference of the disk, and through it some force is applied. Naturally, this tangential action would cause the disk to rotate. We are required to find the torque when the disc starts rotating about its central axis. The details provided to us include:
Radius of the disc is
Force acting is
Moment of inertia is
We know that the torque can be calculated as:
Substituting the given values in the above equation, we get:
Hence, the amount of torque acting along the central axis of the provided disc is 5 Nm.
Note
Moment of inertia is helpful in determining the angular momentum of a rotating body having some mass. In this question, we did not need to use this property because the axis about which the moment of inertia was given coincided with the axis about which we were required to find the torque.
Torque is the force that is responsible for making an object rotate about an axis. Depending on the position of the axis and the distance of the force acting, the value of torque may vary.
Formula used:
Complete step by step answer
In this question, we are provided with a circular disc. A string is attached on the circumference of the disk, and through it some force is applied. Naturally, this tangential action would cause the disk to rotate. We are required to find the torque when the disc starts rotating about its central axis. The details provided to us include:
Radius of the disc is
Force acting is
Moment of inertia is
We know that the torque can be calculated as:

Substituting the given values in the above equation, we get:
Hence, the amount of torque acting along the central axis of the provided disc is 5 Nm.
Note
Moment of inertia is helpful in determining the angular momentum of a rotating body having some mass. In this question, we did not need to use this property because the axis about which the moment of inertia was given coincided with the axis about which we were required to find the torque.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




