
The length of the intercept on y-axis, by circle whose diameter is the line joining the points
A)
B)
C)
D)None of these.
Answer
519k+ views
1 likes
Hint: First find the midpoint of 2 given points which will in turn become the center of the circle as 2 points are the endpoint of diameter. Find distance between 2 points center, any point to get the radius. As you know center and radius find the equation of circle. The y-intercept of the circle in the form of
Complete step by step solution:
The two given points of diameter are written as follows:
Let the center of the circle be
By above we can say the following statements:
x coordinate of the point denoted by A is given by -4.
x coordinate of the point denoted by B is given by 12.
x coordinate of the point denoted by O is given by x.
y coordinate of the point denoted by A is given by 3.
y coordinate of the point denoted by B is given by -1.
y coordinate of the point denoted by o is given by y.
The point O is the midpoint of points A, B.
The x coordinate of O is average of x coordinates of A, B, we get:
By simplifying we get the value of x to be as:
The y coordinate of O is average of y coordinates of A, B, we get:
By simplifying we get the value of y to be as:
So, the center of circle is given by point O
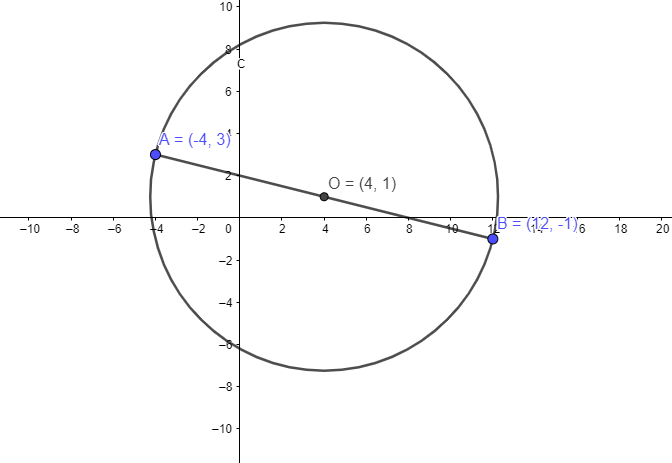
The radius of the circle can be denoted as OA.
The distance between two points (a, b) (c, d) is d, can be given by:
By substituting the values, we can write value of radius as:
Radius = distance between
By simplifying the above equation we can get value of radius as:
Radius
Center
If center is (g, f) and radius r, we get equation as:
By substituting the values, we get it as:
By substituting
By simplifying the above equation, we get final equation as:
By comparing it to
We know he y intercept given by:
By substituting f, c values, we get it as:
52 can be written as
y intercept
Therefore, option (c) is the correct answer.
Note: Be careful while getting the center as the whole equation of circle depends on that point. Don’t confuse between x, y coordinates. Alternate method is to substitute
Complete step by step solution:
The two given points of diameter are written as follows:
Let the center of the circle be
By above we can say the following statements:
x coordinate of the point denoted by A is given by -4.
x coordinate of the point denoted by B is given by 12.
x coordinate of the point denoted by O is given by x.
y coordinate of the point denoted by A is given by 3.
y coordinate of the point denoted by B is given by -1.
y coordinate of the point denoted by o is given by y.
The point O is the midpoint of points A, B.
The x coordinate of O is average of x coordinates of A, B, we get:
By simplifying we get the value of x to be as:
The y coordinate of O is average of y coordinates of A, B, we get:
By simplifying we get the value of y to be as:
So, the center of circle is given by point O

The radius of the circle can be denoted as OA.
The distance between two points (a, b) (c, d) is d, can be given by:
By substituting the values, we can write value of radius as:
Radius = distance between
By simplifying the above equation we can get value of radius as:
Radius
Center
If center is (g, f) and radius r, we get equation as:
By substituting the values, we get it as:
By substituting
By simplifying the above equation, we get final equation as:
By comparing it to
We know he y intercept given by:
By substituting f, c values, we get it as:
52 can be written as
y intercept
Therefore, option (c) is the correct answer.
Note: Be careful while getting the center as the whole equation of circle depends on that point. Don’t confuse between x, y coordinates. Alternate method is to substitute
Latest Vedantu courses for you
Grade 11 Science PCM | CBSE | SCHOOL | English
CBSE (2025-26)
School Full course for CBSE students
₹41,848 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
How much is 23 kg in pounds class 11 chemistry CBSE

How do I get the molar mass of urea class 11 chemistry CBSE

Name the Largest and the Smallest Cell in the Human Body ?

Where can free central placentation be seen class 11 biology CBSE

What is the molecular weight of NaOH class 11 chemistry CBSE

What is the chemical name of Iron class 11 chemistry CBSE




