
The kinetic energy
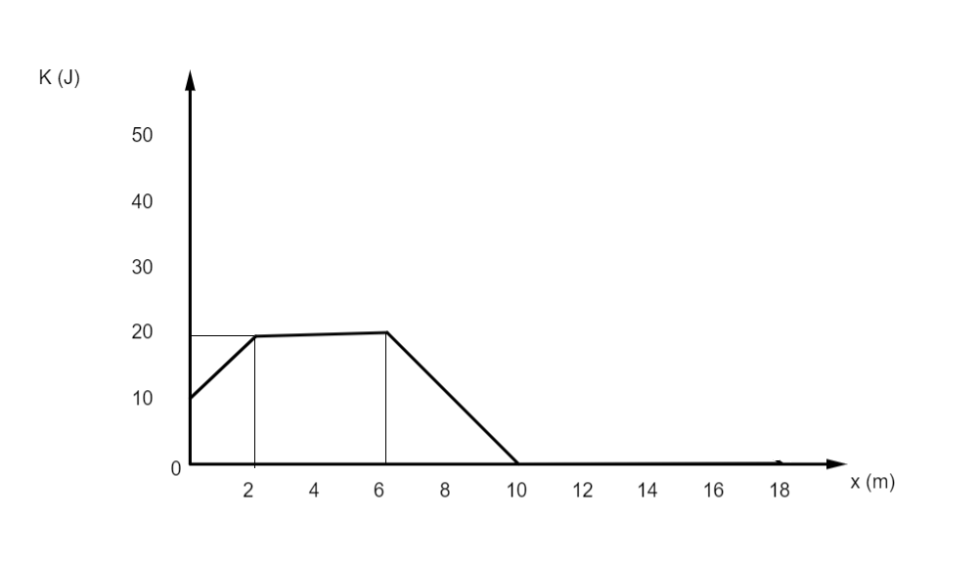
The magnitude of force acting on particle

Answer
500.1k+ views
Hint: Here, the given graph is plotted between kinetic energy and displacement. From the graph, it is clear that kinetic energy is changing with respect to the displacement. By work-energy theorem, change in kinetic energy is equal to the work done. From this concept, we can find force, as work done is equal to the dot product of force and displacement.
Formula used:
Complete answer:
From the given plot between kinetic energy and displacement, it is clear that when the displacement is
where
Let this be equation 1.
We also know that work done is equal to the dot product of force and displacement. Therefore, we have
where
Let this be equation 2.
From equation 1 and equation 2, we have
Let this be equation 3.
Now, from the given graph, it is clear that
Hence, by finding the slope, we can find the force acting on a particular point.
Clearly, force can be expressed as
where
Let this be expression 4.
Solving expression 4 by substituting the values given in graph, we have
Hence, force acting from
Therefore, at
The correct answer is option
Note:
In the equation
Formula used:
Complete answer:

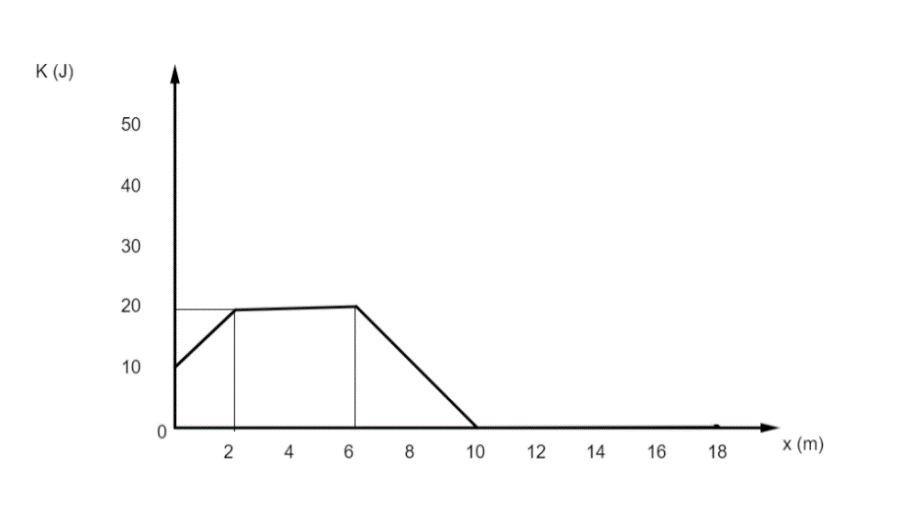
From the given plot between kinetic energy and displacement, it is clear that when the displacement is
where
Let this be equation 1.
We also know that work done is equal to the dot product of force and displacement. Therefore, we have
where
Let this be equation 2.
From equation 1 and equation 2, we have
Let this be equation 3.
Now, from the given graph, it is clear that
Hence, by finding the slope, we can find the force acting on a particular point.
Clearly, force can be expressed as
where
Let this be expression 4.
Solving expression 4 by substituting the values given in graph, we have
Hence, force acting from
Therefore, at
The correct answer is option
Note:
In the equation
Latest Vedantu courses for you
Grade 8 | CBSE | SCHOOL | English
Vedantu 8 CBSE Pro Course - (2025-26)
School Full course for CBSE students
₹45,300 per year
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

A car travels 100 km at a speed of 60 kmh and returns class 11 physics CBSE

What is 1s 2s 2p 3s 3p class 11 chemistry CBSE

In tea plantations and hedge making gardeners trim class 11 biology CBSE

A difference between diffusion and osmosis is a A semipermeable class 11 chemistry CBSE




