
The internal center of similitude of two circles
Answer
519k+ views
Hint: We know that if
Complete step by step solution:
We know that if the internal centre of similitude of two circles
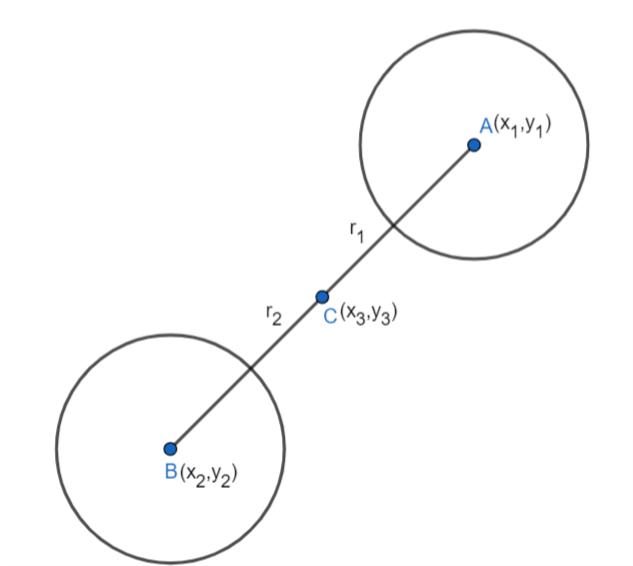
In the question, we were given two circles
Now let us compare
We get
Now let us compare
We get
We know that the internal centre of similitude divides the line joining the centre in the ratio
So, we get the centre of
In the similar manner, we get centre of
So, it is clear that
We know that if
By using this concept, we get
In the similar way, we get
From equation (7) and equation (8), we get the coordinates of internal centre of similitude is
Hence, option A is correct.
Note: Some students have a misconception that if
Complete step by step solution:
We know that if the internal centre of similitude of two circles

In the question, we were given two circles
Now let us compare
We get
Now let us compare
We get
We know that the internal centre of similitude divides the line joining the centre in the ratio
So, we get the centre of
In the similar manner, we get centre of
So, it is clear that
We know that if
By using this concept, we get
In the similar way, we get
From equation (7) and equation (8), we get the coordinates of internal centre of similitude is
Hence, option A is correct.
Note: Some students have a misconception that if
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




