
The interior of a building is in the form of a right circular cylinder of a diameter 4.2m and height 4 m, surmounted by a cone. The vertical height of the cone is 2.1m. Find the outer surface area and volume of the building. (Use π = 22/7)
Answer
480.9k+ views
1 likes
Hint: Outer surface area of the building is the sum of Curved surface area of the cylinder and the curved surface area of the cone because the top and bases of the cylinder and cone are not considered as the outer parts of the building. Volume of the building is the sum of the volume of the cylinder and cone. Find the volumes and curved surface areas using the below mentioned formulas.
Formulas used:
Curved surface area and volume of a cylinder are
Curved surface area and volume of a cone are
Complete step-by-step answer:
We are given to find the outer surface area and volume of the building with base as a cylinder and a surmounted cone.
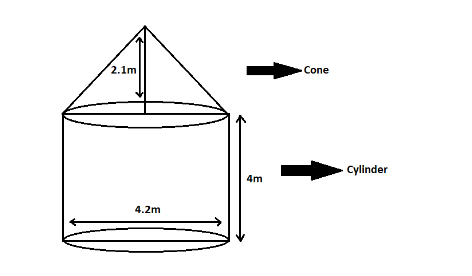
Radius is half the diameter; here the radius is half of 4.2m which is 2.1 m.
Outer surface area = Curved surface area of (Cylinder + Cone)
Curved surface area of the Cylinder is
To find the curved surface area of the cone, we need to find the length of its slant height.
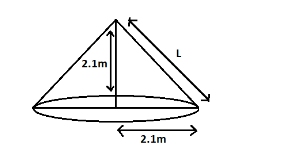
We can find the slant height using the Pythagoras theorem.
Curved Surface area of the cone is
Outer surface area of the building is
Next we have to find the volume of the building.
Volume of the cylinder is
Volume of the cone is
So, the correct answer is “
Note: Pythagoras theorem can be used only in right triangles, according to it, hypotenuse square is equal to the sum of the squares of its adjacent sides. And do not confuse vertical height with slant height in a cone. To solve this type of questions, we just need to know the formulas of their volumes and curved surface areas and if required total surface areas. Total surface area is different from curved surface area.
Formulas used:
Curved surface area and volume of a cylinder are
Curved surface area and volume of a cone are
Complete step-by-step answer:
We are given to find the outer surface area and volume of the building with base as a cylinder and a surmounted cone.

Radius is half the diameter; here the radius is half of 4.2m which is 2.1 m.
Outer surface area = Curved surface area of (Cylinder + Cone)
Curved surface area of the Cylinder is
To find the curved surface area of the cone, we need to find the length of its slant height.

We can find the slant height using the Pythagoras theorem.
Curved Surface area of the cone is
Outer surface area of the building is
Next we have to find the volume of the building.
Volume of the cylinder is
Volume of the cone is
So, the correct answer is “
Note: Pythagoras theorem can be used only in right triangles, according to it, hypotenuse square is equal to the sum of the squares of its adjacent sides. And do not confuse vertical height with slant height in a cone. To solve this type of questions, we just need to know the formulas of their volumes and curved surface areas and if required total surface areas. Total surface area is different from curved surface area.
Recently Updated Pages
Master Class 9 General Knowledge: Engaging Questions & Answers for Success

Master Class 9 English: Engaging Questions & Answers for Success

Master Class 9 Science: Engaging Questions & Answers for Success

Master Class 9 Social Science: Engaging Questions & Answers for Success

Master Class 9 Maths: Engaging Questions & Answers for Success

Class 9 Question and Answer - Your Ultimate Solutions Guide

Trending doubts
What is the difference between Atleast and Atmost in class 9 maths CBSE

What was the capital of the king Kharavela of Kalinga class 9 social science CBSE

What is pollution? How many types of pollution? Define it

Describe the factors why Mumbai is called the Manchester class 9 social science CBSE

Write an essay in about 150 200 words on the following class 9 english CBSE

Write the 6 fundamental rights of India and explain in detail




