
The graph of the given equation
A) Has its lowest point(-1,9)
A. Has its lowest point(1,1)
B. Has its lowest point at (-1,1)
C. Has its highest point at (-1,9)
D. Has its highest point at (-1,1)
Answer
456k+ views
Hint: The given questions asks to find the either the highest or the lowest point of the given equation. In order to do that, we will at first figure out the shape of the equation. As we can see that it is a quadratic equation, where coefficient of x is not zero, thus it is safe that the equation is of parabola. And then to To find the lowest or the highest point of the parabola we find the vertex of the parabola.
Complete step by step answer:
Firstly let us learn about parabolas before dwelling into the question.
When you see a quadratic equation of form
Now see, if the equation is of format
And if the equation is of the form
now, talking about the question in hand,
we have given equation,
We can see that the equation is of the first format, therefore, the given parabola opens vertically.
The vertex of the parabola is given by,
Therefore,
If
Therefore, the parabola has its lowest point at,
whose graph is given below,
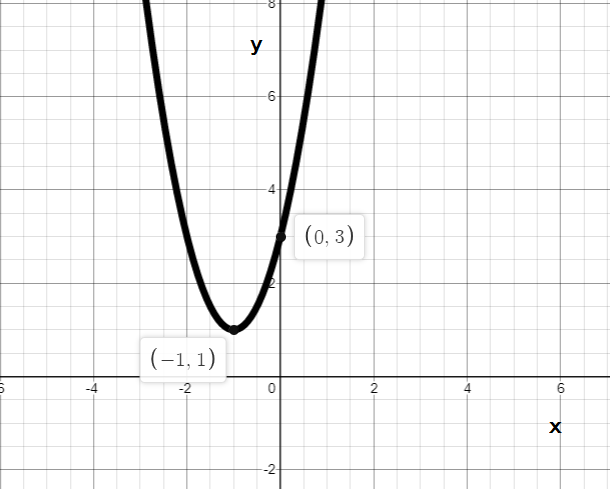
So, the correct answer is Option B.
Note: The equations
Complete step by step answer:
Firstly let us learn about parabolas before dwelling into the question.
When you see a quadratic equation of form
Now see, if the equation is of format
And if the equation is of the form
now, talking about the question in hand,
we have given equation,
We can see that the equation is of the first format, therefore, the given parabola opens vertically.
The vertex of the parabola is given by,
Therefore,
If
Therefore, the parabola has its lowest point at,
whose graph is given below,

So, the correct answer is Option B.
Note: The equations
Recently Updated Pages
Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Social Science: Engaging Questions & Answers for Success

Master Class 11 Physics: Engaging Questions & Answers for Success

Master Class 11 Biology: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

State and prove Bernoullis theorem class 11 physics CBSE

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells




