
The frame of brass plate of an outer door design has area 1.60
Answer
505.2k+ views
Hint: The shear force acts along the back and front of the brass plate and causes a displacement in the bottom part so that one face is displaced by 0.32 mm with respect to another face of the brass plate.
Formula used:
The modulus of rigidity is given by:
Complete answer:
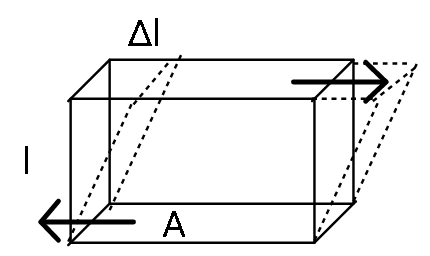
The important point to solve this question is the geometry of the problem. We have to know which faces of our cuboidal brass plate suffer the tangential stresses. Here, we have a horizontal version of the plate in the diagram i.e., to consider the actual situation, one should rotate this picture by a right angle so that the plate stands vertically. Anyways, the shear acts in two opposite directions as shown which will be front and the back end of the plate if it were vertical.
Modulus of rigidity for share modulus is the ratio of tangential stress acting on the body to shear strain that the body undergoes by the stress. The formula for modulus of rigidity will give us:
Now, for brass,
Substituting these values in the formula, we write:
Therefore, this is the amount of parallel force that was applied to create a lateral displacement of 0.32 mm.
Note:
It might be a little confusing to find out the faces that undergo the parallel force action. In this case one can plainly keep all the given values in the simple formula for modulus of rigidity and obtain the answer but one does not get this lucky all the time so when should pay some attention and observe the geometry of the problem carefully.
Formula used:
The modulus of rigidity is given by:
Complete answer:
The important point to solve this question is the geometry of the problem. We have to know which faces of our cuboidal brass plate suffer the tangential stresses. Here, we have a horizontal version of the plate in the diagram i.e., to consider the actual situation, one should rotate this picture by a right angle so that the plate stands vertically. Anyways, the shear acts in two opposite directions as shown which will be front and the back end of the plate if it were vertical.

Modulus of rigidity for share modulus is the ratio of tangential stress acting on the body to shear strain that the body undergoes by the stress. The formula for modulus of rigidity will give us:
Now, for brass,
Substituting these values in the formula, we write:
Therefore, this is the amount of parallel force that was applied to create a lateral displacement of 0.32 mm.
Note:
It might be a little confusing to find out the faces that undergo the parallel force action. In this case one can plainly keep all the given values in the simple formula for modulus of rigidity and obtain the answer but one does not get this lucky all the time so when should pay some attention and observe the geometry of the problem carefully.
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

1 Quintal is equal to a 110 kg b 10 kg c 100kg d 1000 class 11 physics CBSE




