Question

The excess pressure across a soap bubble of radius r is $p = \dfrac{{4\sigma }}{r}$, where $\sigma $ the surface tension of soap solution is. What is the excess pressure across an air bubble of the same radius r formed inside a container of soap solution?
$
  (a){\text{ }}\dfrac{\sigma }{r} \\
  (b){\text{ }}\dfrac{{2\sigma }}{r} \\
  (c){\text{ }}\dfrac{{4\sigma }}{r} \\
  (d){\text{ none of these}} \\
 $

Answer 1

Hint – In this question use the concept that a soap bubble has two surfaces in air but an air bubble has only one surface in soap solution, due to this the surface tension of the air bubble is half of the surface tension of soap bubble. This will help get the right excess pressure.

Complete step-by-step solution -

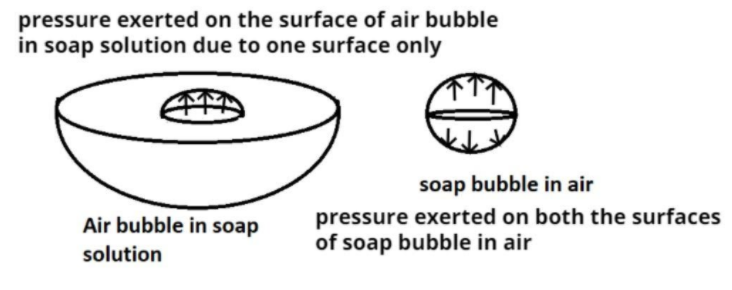
Given data:
Excess pressure on the soap bubble of radius r, $p = \dfrac{{4\sigma }}{r}$, where $\sigma $ is surface tension of the soap solution.
Now as we know that soap bubbles have two surfaces in air but air bubbles have only one surface in soap solution.
So the surface tension on the air bubble is half of the soap bubble.
Therefore surface tension on air bubble = $\dfrac{\sigma }{2}$
As from the given formula pressure is directly proportional to surface tension.
Let the excess pressure on the air bubble be (${p_1}$).
And it is given that the radius of both the bubbles is equal.
$ \Rightarrow {p_1} = \dfrac{4}{r}.\dfrac{\sigma }{2} = \dfrac{{2\sigma }}{r}$
So this is the required answer.
Hence option (B) is the correct answer.

Note – Surface tension is the tension of the surface film of a liquid caused by the attraction of the particles in the surface layer by the bulk of the liquid. In general the molecules beneath the surface try to drag the molecules present on the surface down so as to attain minimum surface tension.