
State Newton’s Law of gravitation expresses it in vector form.
Answer
496.2k+ views
Hint: The Newton’s Law of gravitation is about the force of attraction that exists between all bodies in the universe. It tells us about how the force is dependent on certain parameters like mass, distance etc. The vector form is derived by expressing the vectors in a plane and then using their relations with each other.
Formula used:
Complete step-by-step answer:
Newton’s Law of Gravitation is a universal law which states that every particle in the universe attracts other particles with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between the centers of the two particles.
Let a particle of mass
On removing the proportionality sign, we get,
Let us consider two point masses A and B of mass

Here,
By Newton’s Law of Gravitation, we know that,
As
As we know,
From eq (1) and eq (2), we have,
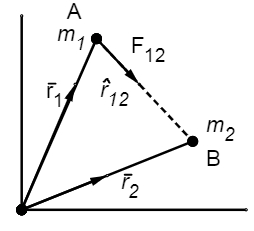
From the above figure,
Substituting eq (3) in eq (1)
To summarize, Newton’s Law of gravitation states that every particle in the universe attracts every other particle with the force such that,
Note: Gravitational forces on two given bodies are opposite in direction but equal in magnitude. Vectors are quantities which have magnitude as well as direction. The unit vector is given by
Formula used:
Complete step-by-step answer:
Newton’s Law of Gravitation is a universal law which states that every particle in the universe attracts other particles with a force that is directly proportional to the product of their masses and inversely proportional to the square of the distance between the centers of the two particles.
Let a particle of mass
On removing the proportionality sign, we get,
Let us consider two point masses A and B of mass

Here,
By Newton’s Law of Gravitation, we know that,
As
As we know,
From eq (1) and eq (2), we have,

From the above figure,
Substituting eq (3) in eq (1)
To summarize, Newton’s Law of gravitation states that every particle in the universe attracts every other particle with the force such that,
Note: Gravitational forces on two given bodies are opposite in direction but equal in magnitude. Vectors are quantities which have magnitude as well as direction. The unit vector is given by
Recently Updated Pages
Master Class 11 Business Studies: Engaging Questions & Answers for Success

Master Class 11 Economics: Engaging Questions & Answers for Success

Master Class 11 Accountancy: Engaging Questions & Answers for Success

Master Class 11 Computer Science: Engaging Questions & Answers for Success

Master Class 11 English: Engaging Questions & Answers for Success

Master Class 11 Maths: Engaging Questions & Answers for Success

Trending doubts
Which one is a true fish A Jellyfish B Starfish C Dogfish class 11 biology CBSE

The flightless birds Rhea Kiwi and Emu respectively class 11 biology CBSE

Difference Between Prokaryotic Cells and Eukaryotic Cells

1 ton equals to A 100 kg B 1000 kg C 10 kg D 10000 class 11 physics CBSE

One Metric ton is equal to kg A 10000 B 1000 C 100 class 11 physics CBSE

How much is 23 kg in pounds class 11 chemistry CBSE




